by Maryam Koozehkanani, Western University
 This page shares resources to help us see math, art and coding, individually and in combination, in new light.
This page shares resources to help us see math, art and coding, individually and in combination, in new light.
We organize the collection in 4 categories:
- math + code + art (links to the Web)
- math + story / poem (annotated bibliography)
- math + dance / music (annotated bibliography)
- math + arts theory (annotated bibliography)
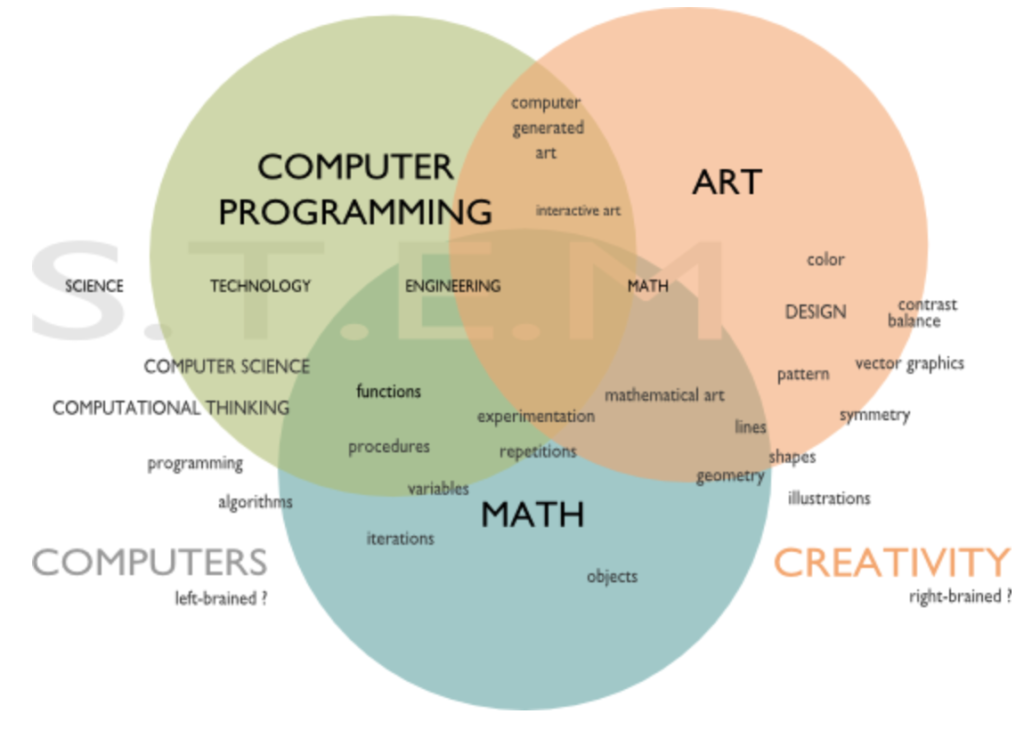
1. MATH + CODE + ART
We have started by identifying publicly available examples where artists, mathematicians, educators and computer scientists use code to create art.
As you study the examples below, you may find it difficult to separate art, math and code, or to see where one starts and the other begins.
Bill Ralph, Mathematics Professor, Brock University
“My work is a personal visualization of patterns and structures emerging from mathematical processes that are simulated by computer programs I design.”
Math art in code
https://www.intmath.com/math-art-code/
This website has six computer-generated works of art that have mathematics at their core.
There is an access to the code and the underlying mathematics of each artwork.
Programming, Math and Art
http://www.computersforcreativity.com/about/programming-art-math
 This site’s content is based on 12 years of teaching experience of Sheena Vaidyanathan, a computer scientist, who is an artist and educator, in the public schools.
This site’s content is based on 12 years of teaching experience of Sheena Vaidyanathan, a computer scientist, who is an artist and educator, in the public schools.
This page explains how art, programming and mathematics are connected. The elements which are in common between art and programming, programming and math, art and math and eventually art, programming and math are mentioned briefly.
Algorithmic Mathematical Art
http://xahlee.info/math/algorithmic_math_art.html
 This website illustrates a collection of algorithmic mathematical artwork.
This website illustrates a collection of algorithmic mathematical artwork.
The differences between artworks and algorithmic ones are explained and it is emphasized that all artworks generated by computers are not necessarily algorithmic.
Tynker coding for kids
https://www.tynker.com/play/math-art/58fe03871c36d137428b459a
In this website there are opportunities for kids to generate visual mathematical artworks by coding.
Generating Art With Code: Doodling, Math and Cornucopias
https://www.coderhood.com/generating-art-code-doodling-math-cornucopias/
The writer, Lorenzo Pasqualis, has two different passions which are art creation and coding. He uses math and coding to generate artistic visions.
Math Artwork
http://www.bugman123.com/Math/
On this page you will find some tessellations, surfaces, and other math stuff along with some basic Mathematica code.
Bridging the gap between art and code
“To UCLA visual artist Casey Reas, writing computer code and programming are not so much technical skills as “thinking” skills that he has managed to apply to artistic expression to great effect.”
“It’s a very interesting and powerful way of thinking that doesn’t have a specific domain,” Reas explained.
Raven Kwok, China
Raven Kwok (aka Guo, Ruiwen) is a visual artist, animator and creative programmer, whose artistic and research interest mainly focus on exploring generative visual aesthetic brought by computer algorithms and software processes.
Joseph Nechvatal, digital artist
Joseph Nechvatal’s Computer Virus Project 2.0 follows along the same lines as previous viral works by Nechvatal in 1992 – works where an unpredictable progressive virus operates on a degradation/transformation of an image. Using a C++ framework, Joseph Nechvatal and his programmer/collaborator Stephane Sikora have brought Nechvatal’s early computer virus project into the realm of artificial life (A-Life) (i.e. into a synthetic system that exhibits behaviors characteristic of natural living systems). With Computer Virus Project 2.0, elements of artificial life have been introduced in that viruses are modeled to be autonomous agents living in/off the image. The project simulates a population of active viruses functioning as an analogy of a viral biological system. Here the host of the virus are the digital files on which the computer-robotic assisted paintings in this show are based. Among the different techniques used here are models that result from embodied artificial intelligence and the paradigm of genetic programming.
The Draftmasters – Part 1
The Draftmasters – I from Daniel Iglesia on Vimeo.
The Draftmasters (Victor Adan and Jeff Snyder), perform live on hacked pen-plotter printers. Using the Manta interface, physical gestures become printer commands. Electromagnetic pickups attached to the printers turn their electrical fields into sound. Daniel Iglesia analyzes their video to create graphics for 3D glasses in real time.
links:
Victor Adan – victoradan.net
Daniel Iglesia – danieliglesia.com
Jeff Snyder – scattershot.org , snyderphonics.com
Columbia Computer Music Center – music.columbia.edu/cmc
Manfred Mohr, Paris France, February 1975
 “The fundamental view that machines should not be considered as a challenge to humanity but, like McLuhan predicted, as an extension of ourselves is the basic philosophy when becoming involved with technology.”
“The fundamental view that machines should not be considered as a challenge to humanity but, like McLuhan predicted, as an extension of ourselves is the basic philosophy when becoming involved with technology.”
“A technology which ‘functions’ has to be integrated in our lives like a physical extension—a necessity of our body and our mind. We are living now in an era of enormous technological transitions, where so many misunderstandings in human machine relationships are created by lack of knowledge and the categorical refusal to learn by most individuals. A quasi mystical fear of an incomprehensible technology is still omnipresent.”
Camille Utterback: Abundance
At night Abundance transforms the San Jose City Hall Plaza and Rotunda into an interactive social space. A video camera mounted on the City Hall captures the movements of people walking below, as a dynamic animation generated in response to this movement is projected onto the cylindrical rotunda. Photo credit: Lane Hartwell Commissioned for the City of San Jose, California by ZER01 – the Arts and Technology Network. Exhibited September 28 – October 6, 2007.
Pascal Dombis: Irrational Geometrics, 2008
When you take a line fragment and give it a stretch, as you do with the string of a bow, the first result is a curve, then a circle and in case you go for it, endlessly, the ultimate artefact is a line again. That series works on the stretch of the line and push the principle of fake straight lines and genuine curves (as if straightened up eventually, out of saturation) to its utter limits.
Deep Art
Turn your photos into art.
We use an algorithm inspired by the human brain. It uses the stylistic elements of one image to draw the content of another. Get your own artwork in just three steps.
Our mission is to provide a novel artistic painting tool that allows everyone to create and share artistic pictures with just a few clicks.
We are five researchers working at the interface of neuroscience and artificial intelligence, based at the University of Tübingen (Germany), École polytechnique fédérale de Lausanne (Switzerland) and Université catholique de Louvain (Belgium).
Try it at https://deepart.io
Can an Artificial Intelligence create Art?
Published on 30 Jun 2016
2. MATH + STORY / POETRY
Hebert, T. p., & Furner, J. M. (1997). Helping high school ability students overcome math anxiety through bibliotherapy. The journal for secondary gifted education.8(4), 164-178.
- Bibliotherapy is a therapeutic, discussion- generating technique which offers educators appropriate affective strategies for dealing with mathematics anxiety in secondary math classrooms so that students achieve success.
- Theme: the article introduces a mathematical book and it’s lesson plan and follow-up discussions.
Callan, R. (2004). Reading+ math= a perfect match. Teaching Pre K – 8, 34(4), 50.
- There are six activities begin with storytelling and can lead your students to find delight in the world of math.
- Theme: Integrating math topics after discussing books can help students make a connection to mathematics in their daily life.
Whitin, Ph. E., & Whitin, D.J. (1997). Ice numbers and beyond: language lessons for the mathematics classroom. language arts, 74 (2), 108-115.
- Using metaphorical language to teach math concepts can enliven student interest and understanding.
- Theme: by postponing teaching the technical vocabulary in mathematics and letting students use their own language art in explaining and describing the mathematics activities, the metaphor and story behind mathematical concepts are developed and student’s perspective come into consideration.
Kliman, M., & Kleiman, G. M. (1992). Life among the giants: writing, mathematics and exploring Gulivers’s world. Language arts, 69 (2),128-136.
- Integrating literature, writing and mathematics based on the story of Gulliver’s travels.
- Theme: activities that use literature and the creating, sharing, and discussing of mathematical writing can support a more active and personal approach to the learning of math.
Borsari, R. Sheedy, J., & Siegel, M. (1990). The power of stories in learning mathematics. Language arts, 67 (2), 174-189.
- The power of story in mathematics class is studied in this article.
- Theme: what is the properties of genuine mathematical stories and how teacher should use the stories in the class. How can shift students and teachers from readers to writers of mathematical story books.
Winorgrad, K., & Higgins, K.M. (1994). Writing, reading, and talking mathematics: one interdisciplinary possibility. the reading teacher, 48(4), 310-318.
- The language arts and mathematics can be integrated as student write, solve, and discuss story problems.
- Theme: allowing student to write mathematical stories and solve their own problems, provide them a friendlier context for learning. The article talked about how can encourage students to write stories for mathematics.
Forbringer, L., Hettinger, A., & Rechert, E. (2016). Using the picture book extra yarn to differentiate commo core math instruction. Young children, 22-28.
- Children’s literature provides an ideal springboard for differentiating mathematics instruction to meet the diverse needs of different students.
- Theme: The good mathematics books allows teachers to develop children’s robust conceptual understanding by engaging them in meaningful problem solving in the context of a delightful story. these books bring up opportunities to address a range of Common Core standards, it provides an excellent vehicle for differentiating instruction so that every child in the class can experience both challenge and success.
Anthony, G., Mclachlan, C., & Limfock, P. R. (2015). Narrative assessment: making mathematics learning visible in early childhood settings. Mathematics education research journal, 27(3), 385-400.
- In this paper, the authors draw on data from some teacher interviews and samples of children’s learning stories to examine how mathematics is made visible within learning stories.
- Theme: Despite appreciating that math was embedded in different context like stories, teachers lack confidence to use these type of math activities and they feel more comfortable to use documents teaching math directly.
Richardson, J. S., & Gross, E. (1997). A read-aloud for mathematics. Journal of adolescent & adult literacy, 40 (6), 492-494.
- “The Colour of Magic” is the first novel in Terry Pratchett’s science fiction/fantasy series, Discworld. Richardson and Gross discuss the novel and language arts and math activities that go along with it.
- Theme: by novels and specific language arts activities students can understand mathematical concepts in the more meaningful ways.
Sandefur, S. J., & Cowan, K. W. (2003). Early childhood math strategies in Mo Willems’s Pigeon book series. Young children, 92-96.
- Mathematical problem solving in the Pigeon books allows teachers and children to gain experience in the five major areas, number and operation, pattern and algebra, measurement, Geometry and data analysis of mathematics in the early childhood classroom.
- Theme: The literature-based activities described here show that mathematical thinking can easily be applied to books that are not written with that specific purpose in mind, that using mathematical thinking to solve problems is enjoyable, and that teachers and children working together can unwrap the gift of using mathematical thinking everywhere.
Courtade, G.R., Lingo, A. S., Karp, K. S., & Whitney, T. (2013). Shared story reading: Teaching mathematics to students with moderate and severe disabilities. Teaching exceptional children, 45 (3), 34-44.
- In this article, it is talking about how to choose the mathematical books and how to implement it for students with some disabilities and how to apply appropriate questions to assess students’ understanding.
- Theme: Using children’s literature to create a context for problem solving can result in meaningful mathematics lessons for students with moderate and severe disabilities.
Jacqueline, H. (1999). Interweaving language and mathematics literacy through a story. Teaching children mathematics, 5(9), 520-524.
- Study the impact of applying one story book and how to implement it in the mathematics class.
- Theme: Questioning and Scaffolding can enhance the application of storybooks considerably.
Kliman, M., & Richard, J. (2015). Writing, sharing, and discussing mathematics stories. The Arithmetic teachers, 40(3), 138-141.
- Students write stories about a familiar situation involving a problem that can be resolved with the aid of mathematics.
- Theme: Student’s writing stories help them 1) identify mathematical situation, 2) make opportunities to write about what they find meaningful and 3) help students communicate mathematically.
Jimenez, B. A., & Kemmery, M. (2013). Building the Early Numeracy Skills of Students with Moderate Intellectual Disability. Education and Training in Autism and Developmental Disabilities, 48 (4), 479-490.
- The research question in the paper is: What is the effect of an early numeracy treatment package which includes story-based math problems, systematic instruction (time delay, least to most prompting systems), graphic organizers, and multiple exemplar training on student’s demonstration of targeted early numeracy skills?
- Theme: Results from this study indicated that students with severe disabilities can learn new academic skills. Due to the new skill attainment by the package of intervention, students will be able to access math curriculum at greater depths of knowledge than previously expected, if taught early numeracy skills with a succinct, personally relevant math curriculum.
Kliman, M. (1993). Integrating mathematics and literature in the elementary classroom. The Arithmetic Teacher, 40(6), 318-321.
- The article discusses the integration of mathematics and literature in the elementary classroom. Use of mathematics to explore; Children’s creation of own stories and drawings; Tips for developing activities and establishing an environment to help incorporate literature into the mathematics class.
- Theme: The math story in which students do not learn the math first and then apply it in a story context, has a more effective impact; In this format students explore the mathematics and the story at the same time. They learn and do math as they explore places, characters and events in the story.
Franz, D. P., & Margaret, P. (2005). Using children’s stories in secondary mathematics. American Secondary Education, 33(2), 20-28.
- This article describes activities based on children’s literature used by pre-service and in-service secondary teachers.
- Theme: Secondary teachers can deepen a students’ understanding or make important connections by using children’s literature in their classrooms.
Nevin, M. L. (1992). A language arts approach to mathematics. Arithmetic teacher, 40(3), 142-146.
- In this article one mathematical story, which is about grouping, is added by mathematical activities. Students use math manipulatives to present the story.
- Theme: Integrated language and mathematics activities can help students make sense of mathematics. These activities by using manipulatives enable students to develop an understanding of concept while building visual images.
Healy, L., & Sinclair, N. (2007). If this is our mathematics, what are our stories? International Journal of computers for mathematical learning. 12 (1), 3-21.
- This paper sets out to examine how narrative modes of thinking play a part in the claiming of mathematical territories as our own, in navigating mathematical landscapes and in conversing with the mathematical beings that inhabit them. It was studied what constitutes the narrative mode, drawing principally on four characteristics identified by Bruner and considering how these characteristics manifest themselves in the activities of mathematicians. Using these characteristics, the authors then analysed several examples with expressive technologies and seek to identify the narrative in the interactions of the learners with different computational microworlds
- Theme: By reflecting on the learners’ stories, the authors highlight how particular features, common across the microworlds—motion, colour, sound and the like—provided the basis for both the physical and psychological grounding of the behaviour of the mathematically constrained computational objects. In this way, students constructed and used narratives that involved situating mathematical activities in familiar contexts, whilst simultaneously expressing these activities in ways which—at least potentially—transcend the particularities of the story told.
Borasi, R., Sheedy, J. R., & Siegel, M. (1990). The power of stories in learning mathematics. Language Arts, 67(2), 174-189.
- This article began and ended with a story.it is explained how to transcend from reading to writing the mathematical books; also, it is said how the reading of stories contribute to mathematics instruction.
- Theme: Mathematical stories presented in this article shows that there is not any distinctions between language (context and value-laden) and mathematics (formal and truth).
Flevares, L. M. &, Schiff, J. R. (2014). Learning mathematics in two dimensions: A review and look ahead at teaching and learning early childhood mathematics with children’s literature. Frontiers in Psychology,5(459), 1-12.
- The article critically reviews the rationales given for the use of picture books in mathematics learning, with a special focus on geometry.
- Theme: there is a need for books based on concepts and children must be exposed to the power and versality, usefulness, and flexibility of the stories. Researches and educators have both the opportunity and responsibility to do so.
Skoumpourdi, Ch., & Mpakopoulou, I. (2011). The prints: A picture book for pre-formal geometry. Early Childhood Educ J, 39, 197-206.
- In the article, a picture book, The Prints, is used as an auxiliary means for helping kindergarten children identify the print of a solid shape. The research questions were: (a) Can kindergarten children identify the origin of the plane figures presented? (b) Can they relate the objects presented to the plane figures presented?
- Theme: by exposing children to the book, they recognised the basic plane figures and their source connecting them with the prints of solids real life objects. they were also able to give appropriate examples of plane figures from their every day lives.
Shatzer, J. (2008). Picture book power: Connecting children’s literature and mathematics. The Reading Teacher, 61(8), 649-653.
- The article starts with one mathematical story book, then proceed by analysing the connection between mathematics and stories.
- Theme: teachers can benefit much from the real good picture books by which can help children make math connections in the classroom.
Forbringer, L. L. (2004). The thirteen days of Halloween: using children’s literature to differentiate instruction in the mathematics classroom. Teaching Children Mathematics, 82-90.
- This article describes how the book The Thirteen Days of Halloween (Greene 2000) can be used to teach a variety of mathematical concepts in kindergarten to fourth-grade classrooms.
- Theme: Comprehensive math stories often inspire a variety levels of complexity and they represent a useful tool for teachers trying to meet the diverse needs of students whose readiness for instruction differs widely.
Golden, M. (2007). 10 ways to imbed ELA skills into the math curriculum. The language and Literacy Spectrum,17,47-60.
- There are some students, who are good in mathematical thinking but are unsuccessful in reading comprehension and writing expression. This article suggests by applying ELA skills (English language Arts) can solve this problem.
- Theme: Embedding ELA skills in math lessons can boost students’ ability to express themselves more precisely. One of the skills is using literature in math class. Teachers by using the skills that good readers use when they read, enhance students’ math comprehension and expression.
Pasko, M. (2006). Curriculum connection: linking literature and math. Journal on School Educational Technology, 2(1), 54-58.
- The article explains how literature benefit student in mathematics learning.
- Theme: By integrating math with literature, math learning become joyful and meaningful. More learning takes place for students and their desire to learn more and to refine their knowledge can increase.
Nascimento, M. L. F., & Barco, L. (2016). The man who loved to count and the incredible story of the 35 camels, Journal of Mathematics and the Arts, 10:1-4, 35-43.
- This paper reveals the origin of the best-known story, the riddle of the 35 camels to be divided among three sons, in proportions of one half, one third and one ninth.
- Theme: This riddle is cleverly solved by adding one camel before dividing them amongst the brothers, and then subtracting two camels afterwards. The authors discuss the question of this puzzle’s origins and provide all the solutions to the more general puzzle in which the number of camels and the proportions are permitted to vary.
Glaz, S. (2016). Poems structured by integer sequences, Journal of Mathematics and the Arts, 10:1-4, 44-52.
- This article explores several techniques for constructing poems using properties of sequences of positive integers.
- Theme: Using mathematical concepts to create new types of constraints results in new poetic forms and new sound like Fibonacci, irrational and prime-number poems discussed in this article.
Koss, L. (2015). Differential equations in literature, poetry and film, Journal of Mathematics and the Arts, 9 (1-2), 1-16.
- This paper investigates how differential equations models have been used to study works in literature, poetry and film.
- Theme: Differential equation model (DE model) can provide a different lens for students with strong mathematical backgrounds to approach other disciplines. Similarly, investigating topics from the arts and humanities can help students evaluate strengths or weaknesses in a mathematical model by challenging them to use a differential equation on material that is less familiar than what appears in typical textbook homework problems.
Aharoni, R. (2014). Mathematics, poetry and beauty, Journal of Mathematics and the Arts, 8(1-2), 5-12.
- This article answered this question: What is the common mechanism in mathematics and poetry that creates beauty in such a similar way.
- Theme: Two common techniques can generate beauty in both poems and mathematics: displacement and unexpected twists. The role of displacement in mathematics is the change of perspective is to cast things in a new light. A twist in a mathematical problem can create a new way of looking at a problem, that renders it beautifully transparentand simple.
Grosholz, E. R. (2014). Great circles: the analysis of a concept in mathematics and poetry, Journal of Mathematics and the Arts, 8 (1-2), 24-30.
- Then authors traced the uses of circles in poems by Marlowe, Shakespeare and Keats.
- Theme: What the authors discovered ‘inside’ the circle there is of course quite different (a devil, a planet, a sleeping girl), for what the poet seeks are really conditions of the meaningfulness (intelligibility) of human life. The notion of containment and of intelligibility changes as moving from the investigation of mathematical problems to that of problematic human beings. Still, the circle remains as part of experience and part of best conceptualization of the natural world.
Grumman, B. (2014). Visiomathematical poetry, the triply-expressive poetry, Journal of Mathematics and the Arts, 8(1-2), 31-37.
- The author comment on the metaphor, beginning with its conventional use in wholly verbal poetry using a sonnet by Keats as an example, and then attempt to demonstrate its vitality when employed in ‘plurexpressive poetry’ (or poetry using more than one ‘language’ besides words, such as visual images or mathematical expressions).
- Theme: ‘visiomathematical poems’, which combine words, graphics and mathematics to become ‘triply-expressive’ can be created by the use of metaphor.
Karaali, G. (2014). Can zombies write mathematical poetry? Mathematical poetry as a model for humanistic mathematics, Journal of Mathematics and the Arts, 8(1-2), 38-45.
- In this note, the author share some thoughts on the creative component of mathematics.
- Theme: Mathematics is a creative endeavour, but mathematicians and mathematics instructors often have difficulty convincing others of this fact. In fact, most people who are not already oriented toward mathematics fail to notice that mathematics is a perfect model for what makes an activity human, as it involves the three main ingredients of what makes our species special: cognition, consciousness and creativity. The author claimed that mathematical poetry can be the ideal ambassador for the efforts to humanize mathematics in the eyes of those who mostly care little for mathematics.
Lesser, L. M. (2014). Mathematical lyrics: noteworthy endeavours in education, Journal of Mathematics and the Arts, 8:1-2, 46-53.
- This paper explores various types of mathematical lyrics and their roles in mathematics education. It also provides resources and strategies for creating such lyrics and for using them in an educational setting.
- Theme: Mathematical lyrics are song lyrics connected to, or inspired by, mathematics or statistics. lyrics can have different roles in classes such as: humanizing mathematics, connecting to history, communication to the real world, reinforcing mathematical thinking process, introducing concepts or terms, aiding recall.
Major, A. (2014). Barbers and big ideas: paradox in math and poetry, Journal of Mathematics and the Arts, 8(1-2), 54-58.
- In this article the author examined paradoxes that have intrigued her and present five poems that have been inspired as a result.
- Theme: The author believes that paradox can be wellspring for both mathematical concepts and poetry.
May, D. P. (2014). Complete graphs in the Rubáiyát, Journal of Mathematics and the Arts, 8(1-2), 59-67.
- In this paper, the author examined connections between the quatrains of the Rub´aiy´at using graph theory.
- Theme: The language of graph theory could be applicable to a poetry. One could also use graphs, or more generally any type of incidence structure, as a template for composing a short collection of poems.
Shmukler, A. & Ziskin, C. (2014). Through the looking glass of history: mathematicians in the land of poetry, Journal of Mathematics and the Arts, 8(1-2), 78-86.
- This article provided a substantial list of mathematicians who wrote poetry from antiquity to the middle of the 20th century. In addition, the authors touched lightly on the similarities and differences between creativity in mathematics and poetry.
- Theme: There are differences between art and mathematics like: math is objective, and poetry is subjective. Poetry is highly depending on the language while math has an international language. Mathematical discovery starting stage is imagination and intuition, then a rigorous check by specialized methods needs to be employed, to reach the goal of ‘deep truth’. As for poetry, both the ‘deep truth’ and the methods for checking that a poem reached its goal are not as clear cut. In spite of these differences, from the psychological point of view, the creative processes in mathematics and poetry have much in common. The mathematician speaks about ‘the intensity and passion involved in mathematical research’, while ‘passion, inspiration and muse’ are well-known characteristics of the poet’s work. In addition, the joy and delight of both mathematician and poet when contemplating the finished product of their creativity are much the same. Furthermore, imagination is an important element for both mathematicians and poems.
Glaz, S. (2011). Poetry inspired by mathematics: a brief journey through history, Journal of Mathematics and the Arts, 5(4), 171-183.
- This article explores one of the many manifestations of the link between mathematics and poetry—the phenomenon of poetry inspired by mathematics.
- Theme: The motivation for writing the poems, their mathematical subjects and their poetic styles, vary through history and from culture to culture. Nowadays, poetry inspired by mathematics is used to shape course content by focusing attention on a particular aspect of the material taught in class and acting as a springboard to initiate class-wide or small group discussions, assignments, or projects based on the poem’s content.
Glaz, S. & Liang, S. (2009). Modelling with poetry in an introductory college algebra course and beyond, Journal of Mathematics and the Arts, 3(3), 123-133.
- The focus of this article is the pedagogical use of poetry in a college course, Introductory College Algebra and Mathematical Modelling, which was designed to prepare students with weak mathematics background for science courses.
- Theme: The poetry projects was used to ease the difficulties students have with the transition between word-problems representing natural phenomena, and the corresponding mathematical models – the equations representing the phenomena. The poetry projects also were used to stimulate classroom participation, develop mathematical intuition, enhance number sense and introduce new concepts in an engaging setting.
Russo, T., & Russo, J. (2018). Narrative-first approach: Teaching mathematics through picture story books. Australian Primary Mathematics Classroom, 23(2), 8+. Retrieved from http://link.galegroup.com.proxy1.lib.uwo.ca/apps/doc/A546025217/AONE?u=lond95336&sid=AONE&xid=0143104b
- The four pillars of student engagement, teacher engagement, breadth of mathematics and depth of mathematics are used to explain the benefits of a narrative-first approach for supporting the integration of mathematics and children’s literature.
- Theme: The authors have attempted to demonstrate how the narrative-first approach can simultaneously engage teachers and students and energise the mathematics classroom, whilst allowing a range of mathematical skills and concepts to be covered across a variety of ability levels.
Heuvel-Panhuizen, M. V. D., & Elia, Ilaida. (2011). Kindergarteners’ performance in length measurement and the effect of picture book reading. ZDM Mathematics Education, 43, 621-635.
This paper addresses: Firstly, kindergartners’ performance in length measurement, the components of their performance and its growth over time; secondly, the possibility to develop kindergartners’ performance in length measurement by reading to them from picture books.
Theme: The authors found a weak but significant effect of reading picture books to children on their general measurement performance. However, this effect was only found for K1 children on the component of holistic visual recognition.
Padula, J. (2004). The role of mathematical fiction in the learning of mathematics in primary school. Australian Primary Mathematics Classroom, 9(2), 8-14.
- This article classifies and describes a selection of mathematical fiction. It also provides some practical activities teachers or parents can use to help make the mathematics more explicit and engaging for their children.
- Theme: The mathematics in picture storybooks can be explicit, implicit, or both. Often mathematics is not the author’s intention, but the ideas cannot be avoided because mathematics is so much a part of our existence. In the classroom, children’s literature can be used as a springboard to mathematical activity (Reeves, 1984).
Russo, J., & Russo, T. (2017a). Harry Potter-inspired mathematics. Teaching Children Mathematics, 24(1), 18–19.
- The authors suggest reading, watching, or just discussing J. K. Rowling’s Harry Potter and the Philosopher’s Stone with your class, and then get students to engage with these associated mathematical problems. The problems cover a diverse range of key mathematical concepts.
- Theme: The authors present mathematical activities associated with the stories based on different grades and schedule them weekly in the article.
Russo, T., & Russo, J. (2018). The narrative-first approach: Room on the broom investigation. Prime Number, 33(2), 10–11.
- The authors present one mathematical story and show how to apply it in classes in different steps.
- Theme: Five steps: shared reading, connecting questions, posing the problem, extension students thinking, and reflective discussion are main steps which can be considered in applying mathematical story books in classes.
Russo, J., & Russo, T. (2017e). Using rich narratives to engage students in mathematics: A narrative-first approach. In R. Seah, M. Horne, J. Ocean, & C. Orellana (Ed.), Proceedings of the 54th Annual Conference of the Mathematics Association of Victoria (pp. 78–84). Melbourne, Australia: MAV
- The outline of the article includes: 1. Introducing the Narrative-First Approach 2. An illustrative example: Fish out of Water 3. Workshop: Create your own tasks. The article contains of different examples of the narrative-first approach compared with typical process-curriculum first approach. How to implement the stories with this approach in different grades with different activities are explained.
- Theme: The authors believe the Narrative first Approach: • Emphasises connectivity of mathematical concepts • Provides a greater focus on the narrative ‘hook’ • Allows for greater flexibility and creativity when planning maths activities (which can be energising and enjoyable for teachers to plan) • Rich tasks allow for development of four proficiencies: understanding, fluency, problem solving and reasoning.
Dietiker, L. (2015). Mathematical story: A metaphor for mathematics curriculum. Educational Studies in Mathematics, 90(3), 285-302.
- More specifically, it describes a metaphor of mathematics curriculum as story and defines and illustrates the mathematical story elements of mathematical characters, action, setting, and plot.
- Theme: Drawn from literary theory, this framework supports the interpretation of mathematics curriculum as art, able to stimulate the imagination and curiosity of students and teachers alike. In doing so, it is argued, this framework offers teachers and other curriculum designers a conceptual tool that can be used to improve the mathematics curriculum offered to students in terms of both logic and aesthetic.
Dietiker, L. (2017). What Mathematics Education Can Learn from Art: The Assumptions, Values, and Vision of Mathematics Education. Journal of Education,195(1), 1 – 10.
- In this article, Dietiker (2017) draw from Eisner’s proposal (Eisner (2002) proposes that educational challenges can be met by applying an artful lens) to consider the assumptions, values, and vision of mathematics education by theorizing mathematics curriculum as an art form.
- Theme: An artful interpretation of mathematics allows both its overall structure and its aesthetic dimension, in textbooks and in the classroom, to be recognized and improved. It provides the opportunity to finally change reality for many students, who are often condemned to mathematical stories that are “the same old thing” (Allen-Fuller et al., 2010, P: 231).
3. MATH + MUSIC / DANCE
Greeley, N., & Theresa, R. O. (1998). Now & then: Dancing in time and space. Mathematics Teaching in the Middle School, 4(3), 192-199.
- Teaching the use of fraction. For example, by dividing or doubling the time signature, the tempo of melody change.
- Theme: by changing the rhythm of music and dance the fraction usages can be taught.
Leah, P. (2007, April). Math meet music. Scholastic DynaMath; New York, 25 (7). 8-9.
- The connection between mathematics and music is presented by the connection between fractions in math and timing in music.
- Theme: mathematics is presented in the music by the fractions music notes take. These fractions are important being in time in music.
Elliot, I. (1998). Music, dance, drama and learning. Teaching Pre K-8,28 (6),36.
- The article talks about how one elementary school use art to help kids master in other disciplines like math and science.
- Theme: One section of the article is about how painting, dance and movement can help kids to create geometric shapes.
Hudakova, J., & Kralova, E. (2016). Creative interdisciplinary math lessons by means of music activities. DE GRUYTER, (11).
- The goal of this paper is to introduce the project includes music activities, games, contests and exercises in accordance with mathematics contents.
- Theme: symmetry is the concept which is defined both in mathematics and music and each understanding (musical or mathematical) reinforce the other ones.
Alain, J. (2008). Dance of the trapezoid. Nea today,26(8).25
- Educators use the power of the arts to teach math and science.
- Theme: activities involved movements like dance and arts like drawing can be incorporated into geometry and trigonometry teaching.
Brodie, I., & Gadanidis, G. (2014). Math Performance. Oame/Aoem gazette. 29-31.
- In this paper, some of the teacher resources available on the Festival website (www.mathFest.ca) are shared for creating math performances that capture the imagination and offer the pleasure of mathematical surprise and insight.
- Theme: Children can learn patterning through song, Orff instruments and dance.
Plett, S., L. (2002). At Fullerton College, Music Brings Math Alive. The Chronicle of Higher Education. 48 (33), A12.
- California’s Fullerton College mathematics professor teaches mathematics with the assistance of a guitar.
- Theme: The harmonic series is defined both in music and mathematics so by music, in particular to this article, playing guitar, can be advantageous in teaching harmonic series in mathematics.
Gesit, k., & Geist, E. A. (2008). Do Re Mi, 1-2-3: that’s how easy math can be: using music to support emergent mathematics. YC young children, 63 (2), 20-25.
- Music can be used for teaching mathematics to infants, toddlers and preschool children.
- Theme: Mathematical activities like patterning and one to one correspondence are easy to link to music. Music has different features like steady beat, rhythm, tempo, volume and melody and they have the potential to have mathematics inside.
Edelson, R. J., & Johnson, G. (2004). Music makes math meaningful. Childhood education, 80(2), 65-70.
- Math and music is a good combination for mathematics education.
- Theme: There are different math-music activities which don’t require musical training or expensive equipment, can be implemented in classes for teaching mathematics.
Church, E. B. (2000&2001). Math & music: the magical connection. Scholastics parent & child, 8 (3), 50-54.
- Math and music unite the two hemisphere of the brain, it’s a powerful force for learning.
- Theme: By exposing children with interconnected activities like math-music ones before learning math terminology directly, they understand the meaning of math and learn the structure of mathematics.
Rogers, G.L. (2004). Interdisciplinary lessons in musical acoustics. The science-math-music connection. Music educators journal, 91(1), 25-30.
- Using common musical instruments and a slinky, teachers can design interdisciplinary activities that teach music, science and math concepts.
- Theme: In the musical acoustic, there are some mathematical aspects which can be introduced to middle and high school students.
Westreich, G. (2002). Dance, mathematics, and rote memorization. Journal of physical education, 73(6), 12-15.
- In the article it is explained how dance and kinesthetics movement can be incorporated in to mathematics class.
- Theme: students’ using dance and kinesthetics movements in expressing mathematics problems builds an understanding of mathematical concepts and provide long-term value.
Cheek, J.M., & Smith, L. R. (1999). Music training and mathematics achievement. Adolescence, 34 (136), 759-761.
- Mathematics scores of eighth graders who had received music instruction were compared according to whether the students were given private lessons. Comparisons also were made between students whose lessons were on the keyboard versus other music lessons.
- Theme: Music training especially with keyboard has a positive impact on the mathematics achievement.
An, S., & Tilman, D. (2014). Mathematics Stories: Preservice Teachers’ Images and Experiences as Learners of Mathematics. Journal of Curriculum theorizing, 39 (2), 20-39.
- This study and others suggest that helping teachers to surface and analyze their mathematics stories can serve as a powerful starting point for in enriching their understanding of what mathematics can be and do.
- Theme: The current study identified the existence of potential opportunities for teachers to facilitate their students’ understanding of mathematics through different types of musical approaches.
Bamberger, J., & Disessa, A. (2003). Music as embodied mathematics: a study of mutually informing affinity. International Journal of computers for mathematical learning, 8 (2), 123-160.
- The argument examined in this paper is that music can become a learning context in which basic mathematical ideas can be elicited and perceived as relevant and important.
- Theme: Perhaps the most general aspect of the affinity between mathematics and music might be the perception and articulate study of patterns. Pursuing this agenda within music might encourage children to become intrigued with patterns in other domains as well.
Jessop, S. (2017). The Historical Connection of Fourier analysis to Music. Mathematics enthusiast, 14 (1,2&3), 77-100.
- The connection between the Fourier series and music is studied in the article.
- Theme: Fourier series does different functions in real life. It added numerous contributions in music and also it is the mathematical base of the encrypting music onto CDs.
Trikoupis, A. (2011). Multidimensional artistic education: the mathematical perspective of music composition. Indicative analysis of applications in the work Jalons by Iannis Xenakis. Review of Artistic Education,1(2), 87-95.
- The article explained the analysis of the work which demonstrates one composer’s mathematical compositional thought and how he developed a new musical form.
- Theme: The mathematics education has been proved to be of great help in the artistic creation. The development of one form of music in this paper is clearly based on numerical relations, organized with the help of mathematical structures, their application being finally subjected to the aesthetic criterion of the composer.
Shilling, W. A. (2002). Mathematics, music, and movement: Exploring concepts and connections. Early Childhood Education Journal, 29(3), 179–184.
- In the article there are some music-mathematical activities in which there are opportunities for children to build understanding not only of time-based relationship, but also of rhythmic pattern.
- Theme: Mathematical thinking and musical appreciation depend on the mathematical patterns embedded in the music. The rhythmical components of music with its accompanying speech afford rich opportunities for exploring mathematical concepts through experiences with beat, meter, duration of sounds, rhythmic patterns, and tempo.
Vaughn, K. (2000). Music and mathematics: modest support for the oft-claimed relationship. Journal of Aesthetic Education, 34 (3/4), 149-166.
- The article is the report of three meta-analyses investigating the relationship of music and mathematics.
- Theme: Students with music background showed higher achievement in mathematics.
An, S., & Tillman, D. (2014). Elementary teachers’ design of arts-based teaching investigating the possibility of developing mathematics-music integrated curriculum. Journal of Curriculum theorizing,30(2), 20-40.
- This study investigated in-service and preservice teachers’ lesson plans on the topic of mathematics and music integration.
- Theme: The music-mathematics integrated teaching strategies identified and examined in the current study is most certainly not a comprehensive typology, but instead includes some of the many possible pedagogical opportunities that teachers can explore as they approach the goal of teaching school subjects like mathematics in innovative ways.
An, S. A., Ma, T., & Capraro, M. M. (2011). Preservice teachers’ beliefs and attitude about teaching and learning mathematics through music: An intervention study. School Science and Mathematics, 111(5), 236-248.
- The present study seeks to investigate the effects of a mathematics–music-integrated intervention activity on preservice teachers’ belief, confidence, motivation, and engagement toward teaching and learning mathematics.
- Theme: the article demonstrates that a mathematics lesson integrated with music has positive effects on preservice teachers’ attitude, beliefs, engagement, and confidence toward mathematical learning and teaching. Furthermore, teaching mathematics integrated with other subjects can improve students’ knowledge in both areas.
Courey, S. J., Balogh, E., Siker, J. R., & Paik, J. (2012). Academic music: music instruction to engage third-grade students in learning basic fraction concepts. Educational Studies in Mathematics, 81 (2), 251-278.
- This study examined the effects of an academic music intervention on conceptual understanding of music notation, fraction symbols, fraction size, and equivalency of third graders from a multicultural, mixed socio-economic public school.
- Theme: this article shows promise for the use of music to teach fraction concepts in the elementary curriculum. There is compelling reason to view music instruction as an integral part of the elementary curriculum, due to its utility in teaching beginning fraction concepts and related fraction computation to elementary students. Furthermore, the intervention appears to be particularly effective for students who are coming to instruction with a lower than average understanding of fractions.
Padula, J. (2009). More about how to teach fractal geometry with music. amt, 65 (1), 37-40.
- In the article the connection between the fractal music and fractal Geometry are explained briefly.
- Theme: In the music, there are some structure like fractal music in which the application of math can be clarified for students.
Jerez, G. D. (1999). Fractals and music: Electronic musician, 108-113.
- This article explores fractals and discuss how they can be employed in musical composition.
- Theme: The algorithms that generate fractals are typically extraordinary simple.
Padula, J. (2005). Fractal music: the mathematics behind ‘Techno’ music. amt, 61 (2), 6-13.
- This article describes the fractal equation and its application to the composition of music.
- Theme: the algorithms that generate fractal images and music are very simple and they are looping: the solution of the equation is repeatedly fed back into itself.
Johnson, G.L., Edelson, R. J. (2003). Integrating music and mathematics in the elementary classroom. Teaching children mathematics, 474-479.
- In the article different music-mathematics integrated activities are introduced.
- Theme: Broad range of mathematics like recognizing, describing and translating patterns can be taught by music. children whose strengths lie in areas other than the logical-mathematical and ones with a limited musical background can be successful with such activities.
An, S., Capraro, M.M., Tillman, D. A. (2013). Elementary teachers integrate music activities into regular mathematics lesson: Effects on students’ mathematical abilities. Journal for Learning through the Arts: A Research Journal on Arts Integration in Schools and Communities, 9 (1), 1-19.
- This article presents exploratory research investigating the way teachers integrate music into their regular mathematics lessons as well as the effects of music-mathematics interdisciplinary lessons on elementary school students’ mathematical abilities of modeling, strategy and application.
- Theme: Many current educators approach the integration of music into mathematics instruction on low-level mathematics content such as counting beats and singing formulas. This study provided an example of how to design and teach mathematics lessons in alternative ways and incorporate higher-order mathematics content.
An, S. A., Kulm, G. O., Ma, T. (2008). The effects of a music composition activity on Chinese students’ attitudes and beliefs towards mathematics: An exploratory study. Journal of Mathematics Education, 1 (1), 96-113.
- This article presents an exploratory research investigating the integration of pop music and statistics lesson as an intervention to promote students’ attitudes and strengthen and extend their beliefs towards mathematics.
- Theme: The results found that students enjoyed learning mathematics in this activity. The implication of this study showed music integrated mathematics lessons gave students a chance to learn mathematics actively with sense making. In our view, the positive results can be attributed to a combination of closely linked factors: (a) used suitable mathematics –music links to arouse students’ interest in pop music and mathematics learning; (b) used graphic notation to create music based on mathematical rules that allowed a deep but perceivable connection between music and mathematics; (c) rewarded students’ achievements highly by sense of achievement in mathematics, the pleasure of enjoying their own music played by piano and the recognition of peers; (d) deigned and provided mathematics tasks based on students’ unique music works.
Fernandez, R. Ch., Gracia, S. R., Fernandez, A. Ch. (2017). Art, science and magic: music and math the classroom. TEEM,1-5.
- In order to verify if music instruction or lessons benefit the study of mathematics, a research study was conducted on 68 4th-grade students enrolled in a Spanish primary school.
- Theme: The results of this research study, have determined that the relationship between music and mathematics has many benefits, and if they are worked on together, can contribute to optimizing learning of both subjects.
Burack, J. (2005). Unity mind and music: Shaw’s vision continues. The American Music Teacher, 55(1), 84.
- Connection between participation and math ability is explained briefly.
- Theme: Classic music can warm up the part of brain which works in abstract thinking and it can improve spatial-temporal reasoning which is useful in math activities.
Helsa, Y. Hartono, Y. (2011). Designing reflection and symmetry learning by using math traditional dance in primary school. IndoMS. J.M.E, 2 (1), 79-94.
- This research aims to show how dance can collaborate with concepts of mathematics like reflection and symmetry.
- Theme: There are a lot of significant mathematical ideas that can be found in dance such as symmetry, time and space, combinatorics, rotation, number, geometry, patterns, and also for learning in higher education, such as Graph. This study indicates that the focus is not on mathematics as a ready-made product, but the activities required the initiative and creative of students to make the active of students. Through these activities, students are trained in critical thinking and argumentative, it’s very useful for students to develop their insight and knowledge.
Werner, L. (2001). Changing student attitudes toward math: using dance to teach math. Center for Applied Research & Educational Improvement, University of Minnesota,1-5.
- This paper describes results of a study that sought to answer the question, “How does integrating dance and math in an intense co-teaching model of integration affect student attitudes toward learning math?”
- Theme: the innovative collaborative arts integration process changed student attitudes toward math. In general, the dance/math students either stayed the same or increased their scores on the survey, whereas the non-dance/math students stayed the same or decreased their scores.
Lorelei Koss, L. (2016). Differential equations in music and dance, Journal of Mathematics and the Arts, 10:1-4, 53-64, DOI: 10.1080/17513472.2016.1264050
- This article studies the connection between differential equations and music and dance.
- Theme: Audio or visual files illustrating the material on composition using chaos, guitar performance using the wave equation or choreography based on the pendulum can be used in class to help the students to understand the connections between the mathematical models and music and dance.
Bush, M. R. & Roodman, G. M. (2013). Different partners, different places: mathematics applied to the construction of four-couple folk dances, Journal of Mathematics and the Arts, 7(1), 17-28.
- In our paper, the goal is to study how to design four-couple dances in which all dancers move to new places every time through and have new partners every time through.
- Theme: Explicitly constructing sequences of permutations can be employed to achieve the desired dance structure.
Renesse, C. V. & Ecke, V. (2011). Mathematics and Salsa dancing, Journal of Mathematics and the Arts, 5(1), 17-28.
- In the article the authors investigated Salsa dance positions and dance moves from a mathematical point of view.
- Theme: By a mathematical ‘space of Salsa dance moves’, the authors found new positions, for instance the ‘impossible’ position after four half turns in one direction. In addition, dancers can be more creative using complicated leader turns rather than just follower turns. It was understood moving between positions in a different way: instead of repeating fixed memorized sequences of moves. It was obtained clarity about the limits of turns and positions, i.e. clarity about when dancers really do have no choice but to release a hand or undo a move.
Wing L. Mui, W.L. (2010). Connections between contra dancing and mathematics, Journal of Mathematics and the Arts, 4(1), 13-20.
- In this article, we discuss the basic structure of a contra dance (a traditional American folk-dance form) and highlight several connections between contra dancing and mathematics.
- Theme: Many choreographers and callers have developed mathematical ways to analyse and create dances; In hindsight it seems unavoidable, as there is a strong mathematical framework underlying contra dances. Although most of the topics in this article are abstract in nature and may not be as applicable for dance writers as the more ‘applied’ dancemistry (dance mystery) developed by choreographers themselves, they highlight some connections between contra dancing and some beautiful mathematics that can be physically experienced by many dancers who seek it out every week.
Fenton, W. E. (2009). Teaching permutations through rhythm patterns, Journal of Mathematics and the Arts, 3(3), 143-146.
- This article discussed about rhythm patterns and how it was used to study permutations and cyclic groups.
- Theme: The students were quite successful at learning some of the aspects of permutations. Cycle notation, the action of a permutation on an ordered list, and the composition of permutations were highlights.
Parsley, J. & Soriano, C. T. (2009). Understanding geometry in the dance studio, Journal of Mathematics and the Arts, 3:1, 11-18.
- In this article the connection between dance and geometrical shape, which are platonic solids (regular polyhedron) was studied.
- Theme: Perspective, similarity and dimension are vital concepts which choreographers must utilize in their aesthetic design. By using the kinetic, three-dimensional body as a dynamic construction, math students may peer into a choreographer’s toolbox and discover how dance borrows from geometry.
Toussaint, G.T. (2005). The Euclidean algorithm generates traditional musical rhythms. In Proceedings of Bridges: Mathematical Connections in Art, Music and Science, Banff, Canada, 47–56.
- A new family of musical rhythms is described in this article, called Euclidean rhythms, which are obtained by using Bjorklund’s sequence generation algorithm, which has the same structure as the Euclidean algorithm.
- Theme: It was shown that many rhythms used in world music are Euclidean rhythms. Some of these Euclidean rhythms are also Euclidean strings.
Toussaint, G. T. (2002). A mathematical analysis of African, Brazilian, and Cuban clave rhythms. In Proceedings of BRIDGES: Mathematical Connections in Art, Music and Science, Towson University, Towson, MD ,157–168.
- Several geometric, graph theoretical and combinatorial techniques useful for the teaching, analysis, generation and automated recognition of rhythms are proposed and investigated.
- Theme: It is shown that it is possible with three simple geometric features to automatically classify the rhythms without knowledge of their starting note.
Toussaint, G. T. (2003). Classification and phylogenetic analysis of African ternary rhythm timelines. In Proceedings of BRIDGES: Mathematical Connections in Art, Music and Science, Granada, Spain ,25–36.
- The author in some part of the article use a geometrical method to clarify different rhymes.
- Theme: A useful geometric representation for such cyclic rhythms is obtained by connecting consecutive note locations with edges to form a convex polygon. Such a representation not only enhances visualization for classification but lends itself more readily to geometrical analysis.
Toussaint, G. (2010). Computational geometric aspects of rhythm, melody, and voice-leading. Computational Geometry: Theory and Applications, 43(1), 2-22.
- In this paper several geometric properties of musical rhythms, scales, melodies and voice-leading are analysed from the musicological and mathematical points of view.
- Theme: Several connecting bridges between music theory, musicology, discrete mathematics, statistics, computational biology, computer science, and crystallography are illuminated. The interaction between computational geometry and music yields new insights into the theories of rhythm, melody, and voice-leading.
4. MATH + ARTS THEORY
Hickman, R., & Huckstep, P. (2003). Art and mathematics in education. The Journal of Aesthetic Education, 37(1), 1-12.
- The article analyses the connection between art and mathematics.
- Theme: In conceptual level art and mathematics have similarities in term s of creativity and creation. mathematicians use their creativity to create solution and model.
Stylianou, D. A., & Grzegorczyk, I. (2005). Symmetry in mathematics and art: An exploration of an art venue for mathematics learning. Primus, 15(1),30-44.
- The article explores the connection between art and mathematics in the context of a liberal arts mathematics course. It uses symmetry subject which is an aspect of mathematics linked to art.
- Theme: The freedom for students to choose the means in which to apply the concepts they find most useful in the context of art with which they feel familiar, facilitated the learning of mathematics. Further, familiarity with symmetry concepts allow students to use abstraction as a tool in their artistic creations.
Bier, C. (2010). CarpetMath: exploring mathematical aspects of Turkmen carpets. Journal of Mathematics and the Arts, 4 (1), 29-47.
- Exploring mathematical aspects of Turkmen carpets is the aim of this article.
- Theme: These explorations in the mathematical aspects of Turkmen carpets address various topics within the curriculum and are suggested for different age groups: counting units and fractions (K-5); symmetry and geometry (grades 6–8) and algorithms (high school).
Presmeg, N. (2009). mathematics education research embracing arts and sciences. ZDM Mathematics Education,41,131-141.
- Creativity and rigor are required in all mathematics education research; thus it is argued in this paper, using examples, that characteristics of both the arts and the sciences are implicated in this work.
- Theme: Both the sciences and the arts are inevitably implicated in mathematics education.
Taylor, R., Micolich, A., & Jonas, D. (2002). The construction of Jackson Pollock’s fractal drip paintings. Leonardo, 35(2), 203-207.
- In this paper the authors used a fractal analysis technique to examine the painting process Pollock used to construct his drip paintings.
- Theme: the conclusion is that Pollock used a remarkably systematic method capable of generating intricate patterns that exhibit fractal scaling criteria with precision and consistency.
Growney, J. A. (2008). Mathematics influences poetry. Journal of Mathematics and the Arts, 2(1), 1-7.
- In this paper, the author explores some of those likenesses and describe ways that I see mathematics influencing poetry.
- Theme:1. mathematics and poetry demand similar creativities,2. constraints involving mathematics give poets the opportunity to discover new language.3. mathematics offers precise and vivid imagery for poems.
Francis, G.K. Collins, B. (1992). On knot-spanning surfaces: An illustrated essay on topological art. MIT press, 25(3-4), 313-320.
- This essay the author set forth basic vocabulary for a dialogue between artist and mathematician concerning certain kinds of mathematical art-in particular, the topological sculptures of Brent Collins.
- Theme: In the article it was discovered the true topological identity of the surface of this sculpture and its nonobvious affinity to another well-known piece of mathematical art.
Brooks, M. (2009). Drawing, visualization and young children’s exploration of ‘Big Ideas.’ International Journal of Science Education, 31(3), 319-341.
This paper explores how drawing and visualisation bridges the gap between perception-bound thinking and more abstract, symbolical thinking.
- Theme: The article has also demonstrated that children are able to absorb information from the contexts in which they work and to assimilate and transform new ideas through their drawings.
- Drawing can assist young children’s interactions and competencies with spatial visualisations, interpretations, orientations and relations. When young children are able to create visual representations of their ideas they are more able to work at a metacognitive level.
Sakkal, M. (2018). Intersecting squares: applied geometry in the architecture of Timurid Samarkand, Journal of Mathematics and the Arts, 12:2-3, 65-95.
- This paper deals with three subjects: Islamic art and architecture, geometry and mathematics, and Persianate culture in Iran and Central Asia.
- Theme: Analyzing the DS (double square) patterns as a representative type for the class of Square Kufic calligraphy on the walls of Bibi Khanum mosque reveals an admirable richness of compositions. The detailed analysis and exploration of the DS is likely to yield useful information when applied to various pattern generating series as expressed in Bibi Khanum mosque and other Timurid monuments. As presented in this paper, the range of possibilities for intersecting square grids is also of mathematical interest with applications in contemporary design.
Webster, P. (2018) Infinity Flower IV, Journal of Mathematics and the Arts, 12:2-3, 111-113.
- The author focused on blending traditional Islamic motifs with polyhedra and fractals.
- Theme: My general process for creating the works in this series is a method of my own creation for taking traditional Islamic geometric motifs and arranging them into fractal patterns. The results are distinctly Islamic in flavour but with a modern twist. One of the primary outputs of this investigation is the ongoing series of two-dimensional works called Fractal Islamic Patterns.
Bonner, J. (2018). Doing the Jitterbug with Islamic geometric patterns, Journal of Mathematics and the Arts, 12:2-3, 128-143.
- Vertex-to-vertex polyhedral jitterbug transformations provide for the creation of polyhedra with specific angular conditions at the vertices that facilitate regions of local symmetry that are atypical of the spherical geometry associated with the Platonic and Archimedean polyhedra.
- Theme: By the author’s working with applying Islamic geometric patterns to the sphere, it has become clear that jitterbug polyhedra provide unlimited potential for unusual, yet beautiful spherical patterns.
Araújo, A. B. (2018). Ruler, compass, and nail: constructing a total spherical perspective, Journal of Mathematics and the Arts, 12:2-3.
- The main purpose of this work is to offer a method the artist can use for drawing total spherical perspectives like those of, by simple ruler and compass constructions.
- Theme: the author established a general setup for anamorphosis and central perspective, define total spherical perspective within this framework, study its topology, and show how to solve it with simple instruments. We consider its uses both in freehand drawing and in computer visualization, and its relation to the problem of reflection on a sphere.
Sugihara, K. (2018). Topology-disturbing objects: a new class of 3D optical illusion, Journal of Mathematics and the Arts, 12:1, 2-18.
- A new class of objects, called topology-disturbing objects, appear to be separated when seen from one viewpoint while they appear to be intersecting when seen from the other viewpoint is presented in this article.
- Theme: Topology-disturbing objects this cannot happen physically, but due to the phenomenon of an optical illusion, it can be perceived to occur. These objects might provide a new resource for arts and entertainment. In the history of arts and entertainment, optical illusions have been used to create the impression of impossibility.
Wu, J. (2018). Folding helical triangle tessellations into light art, Journal of Mathematics and the Arts, 12:1, 19-33.
- This article concerns the artistic and perceptual quality of translucent light transmitted by an origami-inspired paper surface when a light source is placed behind it.
- Theme: In this article, the author as an artist/designer described how he explored the relationships between geometric strategies in helical triangle tessellations and the perceptual quality of illuminated origami design in order to create light art with interesting effects of light gradation.
Schattschneider, D. (2018). Marjorie Rice (16 February 1923–2 July 2017), Journal of Mathematics and the Arts, 12:1, 51-54.
- The author focused on the story life of Marjorie Jeuck Rice, a most unlikely mathematician and her work which was about different type of pentagons.
- Theme: Marjorie’s discoveries were an important contribution to the problem of characterizing convex pentagons that can tile the plane. For her, however, pentagon tilings also provided networks that could underlie intricate Escher-like designs of flowers, butterflies, bees, shells and fish often coloured to show the different orientations of the transformed tiles.
Seaton, K. A. (2017). Sphericons and D-forms: a crocheted connection, Journal of Mathematics and the Arts, 11:4, 187-202.
- Sphericons and D-forms are 3D objects created and described by artists, which have separately received attention in the mathematical literature in the last 15 or so years.
- Theme: The attempt to classify a seamed, crocheted form geometrically led to the observation, which appears not to have been previously made explicit, that these objects are related.
Knoll, E. (2017). Colourwave: some variations on a mathematical schema, Journal of Mathematics and the Arts, 11:4, 203-222.
- In this paper, the author walks the reader through the process that produced a series of art works and points out some of their mathematical properties.
- Theme: Using colour to track paths of traveling elements in permutation algorithms can not only help visualize what is happening from a mathematical perspective, but it also produces aesthetically pleasing configurations that can be the starting point for compelling artwork.
Happersett, S. (2017). Susan Happersett – artist’s statement, Journal of Mathematics and the Arts, 11:4, 240-242.
- The article is about how the author use art to express the inherent beauty in mathematics.
- Theme: The author developed a new type of drawing process based on set theory and the concept of mapping. Art was the authors’ vehicle to express her fascination with mathematics.
Koss, L. (2017). Visual arts, design, and differential equations, Journal of Mathematics and the Arts, 11:3, 129-158.
- This paper is about to connect ideas from differential equations to relevant and interesting material from the arts and humanities. The author examines applications to painting, architecture, string art, banknote engraving, jewellery design, lighting design, and algorithmic art.
- Theme: For teachers, the topics covered in this paper can also serve as a resource to add supplementary material to courses on calculus or ordinary differential equations. In fact, some of the material in this paper could be examined in a calculus course that introduces differential equations. In particular, the exponential decay model in Section 3 is often covered in standard calculus textbooks. Students could verify that the cycloid and the catenary satisfy the differential equations described in Sections 5 and 6 even if the derivations of the models are not covered.
Stoyan, D. (2016). Point process statistics: application to modern and contemporary art and design, Journal of Mathematics and the Arts, 10:1-4, 20-34.
- The paper studies three examples of random patterns which can be textures of many small objects (grains, elements or marks), which are randomly scattered and placed at random positions (‘points’ or ‘germs’), forming interesting structures.
- Theme: This paper has shown statistically that artists are able to produce marked point patterns which can be considered as samples of statistically homogeneous marked point processes and fitted by standard models of spatial statistics.
Wildstrom, D. J. (2015). Design and serial construction of digraph braids, Journal of Mathematics and the Arts, 9 (1-2),17-26.
- This work determined, for a family of braids which are determined by directed graph structures, how these desiderata can be associated with a mathematical property of the underlying directed graphs.
- Theme: With mathematical developments which grew out of the field of knot theory, it became possible to consider braids not only as physical objects, but as mathematical abstractions, and not only mathematicians but also craftspeople have found the systematization of the field of braid design to be a useful asset in their work while mathematical studies have allowed wholly new designs incorporating braids to be developed algorithmically.
Costa, S. M. & Freitas, P. J. (2015). Almada Negreiros and the geometric canon, Journal of Mathematics and the Arts, 9:1-2, 27-36.
- This paper presents a mathematical analysis of a series of geometrical abstract artworks by the Portuguese author Almada Negreiros (1893–1970).
- Theme: The analysis revealed that some of the author’s geometrical constructions were mathematically exact whereas others were approximations. The findings show that, though limited by the self-taught nature of his endeavour (Almada), the mathematical content of these artworks is surprisingly rich.
Åström, A. & Åström, C. (2015). Circular knotworks II: combining pattern No. 295 with Turk’s heads, Journal of Mathematics and the Arts, 9 (3-4), 91-102.
- This article developed the necessary mathematical tools to characterize the broader class of knotworks in order to produce new designs with specific properties. their technique is based on adding layers of a knot called a Turk’s head.
- Theme: The finding of this article, by presenting an equation to calculate the number of strands, increased the usability for knot artists when creating fancywork for this type of knotwork, since a greater variety of parameter settings can be used.
Irvine, V. & Ruskey, F. (2014). Developing a mathematical model for bobbin lace, Journal of Mathematics and the Arts, 8(3-4), 95-110.
- The authors defined a new mathematical model that supports the enumeration and generation of bobbin lace patterns using an intelligent combinatorial search, and which is capable of producing patterns that have never been seen before.
- Theme: By using braid theory and graph theory the model which describe workable patterns was defined in the article. An intelligent combinatorial search algorithm is used to enumerate and exhaustively generate patterns consistent with this model.
Lang, R. J. (2013). A Pajarita Puzzle Cube in papiroflexia, Journal of Mathematics and the Arts, 7:1, 1-16.
- The author presented the development of a modular origami design based upon the Pajarita, a figure from the traditional Spanish paperfolding art. The work is a modular cube decorated with Pajaritas with the colour pattern produced by folding of individual units to be topologically identical but with distinct colour patterns on each. The mathematics of the cube-colouring and unit-colouring problems are analysed. Two unique solutions are found and presented for the easiest possible cube and a solution for the hardest possible cube.
Farris, F. A. (2013). Symmetric yet organic: Fourier series as an artist’s tool, Journal of Mathematics and the Arts, 7(2), 64-82.
- The article explained how to use mathematics (Fourier series) to make a new tool to create images out of the real pictures.
- Theme: New set of tools based on Fourier series can be applied to create images combining the familiar rhythms of symmetry with the textures and colours of photographs. The colours and textures from photographs of natural scenes, combined with carefully chosen symmetrical complex-valued functions, led to a large collection of images that viewers consistently describe as ‘organic’ and ‘like mathematics, but not too much like mathematic.
Bosch, R. & Colley, U. (2013). Figurative mosaics from flexible Truchet tiles, Journal of Mathematics and the Arts, 7(3-4), 122-135.
- This paper described how to modify Truchet’s tiles, Infinity of pleasing designs made by simple set of square tiles which are divided by a diagonal into a white half and a black half. So that a collection of them can be used for halftoning.
- Theme: In this paper it is described how to modify Truchet’s original tiles so that a collection of them can be used for halftoning, the reproduction of user-supplied greyscale target images in pure black and white. It is presented hexagonal variations, a similar scheme for Truchet-like tiles, and an extension that can be applied to all regular and semiregular tilings.
Goldstine, S. & Baker, E. (2012). Building a better bracelet: wallpaper patterns in bead crochet, Journal of Mathematics and the Arts, 6(1), 5-17
- Creating symmetric colour patterns on bead crochet bracelets is challenging because the beads form a continuous spiral along the length of the bracelet, making it difficult to align design motifs uniformly. In this article new technique was devised to overcome this obstacle.
- Theme: In the article a new technique devised for translating bracelet patterns into plane tilings and used this to create bracelets based on wallpaper group symmetries to stunning effect. The authors proved that exactly 13 of the 17 wallpaper groups can be represented by bead crochet bracelets, and it is outlined a general method for designing a bracelet whose planar bead tiling has a given wallpaper symmetry group.
Zheng, X. & Brown, N. S. (2012). Symmetric designs on hexagonal tiles of a hexagonal lattice, Journal of Mathematics and the Arts, 6(1), 19-28.
- In his paper Fernandez utilized the union of several sublattices of a square lattice to generate a design on a square tile of the Cartesian plane such that the design, the tile and the lattice have the same rotational and reflectional symmetries.
- Theme: The authors have developed some methods to efficiently make various designs on a hexagonal tile of a hexagonal lattice such that the designs, the tile and the hexagonal lattice have the same rotational and reflectional symmetries. The methods for a square lattice were transferred to a hexagonal lattice. Furthermore, the authors considered different kinds of generating sets, and used randomization and several adjusting methods to search for symmetric patterns. Some of the resulting figures were exhibited in the article. Based on the experiments the new methods help to obtain symmetric patterns with diverse features.
Cromwell, P. R. (2012). A modular design system based on the Star and Cross pattern, Journal of Mathematics and the Arts, 6(1), 29-42.
- This article has introduced a modular system derived from the Star and Cross pattern. The family of patterns that can be produced by assembling the modules includes many traditional Islamic patterns from Central Asia and Iran. Some of the simpler patterns, including the Star and Cross pattern itself, are pre-Islamic.
- Theme: The earliest Islamic examples of (Central Asian modular design system) CAMS patterns are found among the Seljuk brickwork designs on eleventh and twelfth century minarets. The restriction to 45_ and 90_ angles in the modules may indicate that CAMS was developed in this medium as these are the angles most compatible with working in brick.
Nake, F. (2012). Information Aesthetics: An heroic experiment, Journal of Mathematics and the Arts, 6(2-3), 65-75.
- Information Aesthetics was a short-lived but influential attempt to establish a mathematically rigorous aesthetic theory without subjective elements.
- Theme: The concept of statistical information developed by Shannon can be used as the mathematical basis of an objective measure of aesthetics.
Fisher, G. L. & Mellor, B. (2012). Using tiling theory to generate angle weaves with beads, Journal of Mathematics and the Arts, 6 (4), 141-158.
- In this article, the authors explored beading patterns arising from periodic polygonal tiling of the plane (It is called angle weaves in the article).
- Theme: In the article it was showed that periodic polygonal tilings is merely the tip of the iceberg of all the possible beading designs that can be inspired by mathematical theory of tiling.
Cromwell, P. R. (2012). Analysis of a multilayered geometric pattern from the Friday Mosque in Yazd, Journal of Mathematics and the Arts, 6(4), 159-168.
- The article discussed the design construction of the Friday Mosque in Iran which has geometric structure.
- Theme: The design of the mosque has geometric structure on two levels: at the large scale the panel is divided into compartments by a network of pathways, and on a much smaller scale, these pathways are paved with a star pattern. In this type of 2-level composition, the compartments are usually filled with a different class of ornament, either calligraphy or arabesques. Here, a few compartments do contain white calligraphy on a dark blue ground, but many of them are filled by a continuation of the small-scale pattern of the pathways.
Séquin, C. H. (2012). Topological tori as abstract art, Journal of Mathematics and the Arts, 6(4), 191-209.
- Topologists have known for almost three decades that there are exactly four regular homotopy classes for marked tori immersed in 3D Euclidean space. Instances in different classes cannot be smoothly transformed into one another without introducing tears, creases, or regions of infinite curvature into the torus surface. In this article, a quartet of simple, easy-to-remember representatives for these four classes is depicted to expose this classification to a broader audience.
- Theme: It is shown explicitly how the four models differ from one another, and examples are given of how a more complex immersion of a torus can be transformed into its corresponding representative. These mathematical visualization models are juxtaposed with artistic sculptures employing more classical torus shapes.
Segerman, H. (2011). Fractal graphs by iterated substitution, Journal of Mathematics and the Arts, 5(2), 51-70.
- The authors introduced a generalization of the construction of space filling curves to constructions of fractal graphs by iterated substitution.
- Theme: In the article it was introduced a generalization of the construction of space filling curves to a construction of space filling graphs, for which the steps retain the self similar structure but have greater connectivity.
Liu, Y. & Toussaint, G. T. (2011). The marble frieze patterns of the cathedral of Siena: geometric structure, multi-stable perception and types of repetition, Journal of Mathematics and the Arts, 5(3), 115-127.
- In this article the geometric frieze patterns found on the pavement, walls and ceiling of the Siena Cathedral are analysed in terms of their underlying geometric structure, the optical effects, such as multi-stable perception, that they engender in the viewer and a typology of patterns of repetition.
- Theme: Repetition is a ubiquitous property of nature, science, visual arts, music and life in general. Here repetition is the main instructional feature of the cathedral of Siena.
Puc, S. & Škrekovski, R. (2011). Contour map patterns, Journal of Mathematics and the Arts, 5(3), 129-140.
- In this article, we have presented a simple procedure for constructing aesthetic coloured repeating patterns with the use of contour lines and maps.
- Theme: The process of generating patterns based on the concept of contour lines of maps is first choosing a periodic function of two variables f(x, y) and a corresponding 3D-graph z=f(x, y). The graph of f is then intersected by k prescribed horizontal planes, and contour lines are projected onto the plane z¼0. Finally, contour regions are coloured by distinct colours. In this way the motif of the pattern is created. The periodicity of the two variables x and y of the function f assures repetition of the motif in two independent directions, and therefore the final result is a two-dimensional repetitive pattern.
Åström, A. & Åström, C. (2011). Circular knotworks consisting of pattern no. 295: a mathematical approach, Journal of Mathematics and the Arts, 5(4), 185-197.
- The intention of the article was to present the mathematical characteristics of the circular knotworks and derive equations for calculating the number of strands.
- Theme: It has been shown that the knotworks can be described using only three parameters and those parameters can be used to calculate the number of strands for this type of knotwork. By developing equations and tools to simplify the work for knot artists when constructing fancywork from different knotworks, it can help further promote the cultural heritage of using knotwork as decoration.
Ross, F.& Ross, W. T. (2011). The Jordan curve theorem is non-trivial, Journal of Mathematics and the Arts, 5(4), 213-219.
- The formal mathematical definition of a Jordan curve (a non-self-intersecting continuous loop in the plane) is so simple that one is often lead to the unimaginative view that a Jordan curve is nothing more than a circle or an ellipse. This article introduced and explained new ideas about JCT in terms of art and mathematics.
- Theme: Jordan curve is so much more than a circle or ellipse. It can be jagged at every point or perhaps impossible to visually determine the interior from the exterior or perhaps even of positive area, and, as seen through the artwork in this paper, can lead the viewer through a story. Jordan curves inspire art and, more importantly, help mathematicians make a better case to the students that the JCT is non-trivial – both as a mathematical result and as a work of art.
Liu, Y. & Toussaint, G. (2010). Unravelling Roman mosaic meander patterns: a simple algorithm for their generation, Journal of Mathematics and the Arts, 4(1), 1-11.
- In this article, a geometrical analysis of the meander decorative patterns on a Roman pavement mosaic found at the Romanvilla in Chedworth, UK, is presented.
- Theme: The geometric analysis provided here of this pattern reveals a simple algorithm by which the Romans could have easily constructed this and other such seemingly complex patterns. This algorithm permits the construction of new alternate designs.
Bier, C. (2010). CarpetMath: exploring mathematical aspects of Turkmen carpets, Journal of Mathematics and the Arts, 4(1), 29-47.
- The author explored the mathematical aspects of Turkmen carpets.
- Theme: Islamic art seems to draw upon a shared heritage of Greek geometry and Indian arithmetic. As with Turkmen carpets, patterns in Islamic art also tend to exploit the inherent possibilities for playfulness and ambiguity in relationships among numbers (arithmetic) and shapes (geometry). The mathematical principles of symmetry (a correspondence of points) are always combined with symmetry-breaking (and sometimes with the absence of symmetry), to produce decorative patterns. Islamic craftsmen certainly must have understood and appreciated how such patterns provide aesthetic pleasure, captivating the mind as they please the eye.
Peter R. Cromwell, P. R. (2010). Islamic geometric designs from the Topkapı Scroll I: unusual arrangements of stars, Journal of Mathematics and the Arts, 4(2), 73-85.
- The Topkap| Scroll is an important documentary source for the study of Islamic geometric ornament. Here we give a mathematical analysis of some its exemplary star patterns that illustrate a variety of methods of construction.
- Theme: The practice of producing a design by replicating a template using reflections in its sides restricts the range of symmetry types produced. In particular, the lack of 3-fold symmetry is related to the exclusive use of rectangular templates. By transferring the distributions of stars traditionally seen in a square template to their equivalent arrangements in an equilateral triangle, the author produced new 3-fold designs in the Islamic style.
Luecking, S. (2010). A man and his square: Kasimir Malevich and the visualization of the fourth dimension, Journal of Mathematics and the Arts, 4(2), 87-100.
- The author offered the geometric and aesthetic rationale as to why Russian artist Kasimir Malevich subtitled five of his paintings as ‘Colored Masses in the Fourth Dimension’, even though they seemingly do not differ significantly from his other works with titles such as ‘Self Portrait in Two Dimensions’.
- Theme: The author asserted that Malevich’s paintings could be viewed as actual visualizations of a 4-D geometry and that his notions about geometry in art rely far more on Nikolai Lobachevsky’s concept of pangeometry. In general mathematics and specifically geometry offered formative systems which may or may not possess referents in nature, or even make conventional sense. The value of a fourth dimension was its virtue as a mathematical and artistic conceit, and not its necessary existence or, the beauty of its mathematical descriptions. Geometry and the art it inspired possessed their own existence not predicated on the logic of a physical reality.
Ashton, B. A. (2010). Integrating elements of Frank Lloyd Wright’s architectural and decorative designs in a liberal arts mathematics class, Journal of Mathematics and the Arts, 4(3), 143-161.
- Frank Lloyd Wright’s architectural designs have many aspects that can be creatively integrated in a mathematics class. In this article, the author described examples from the study of symmetry, graph theory and function theory.
- Theme: The visual excitement and hands-on experience that was added to a mathematics course with the topics described, Frank Lloyd Wright’s architectural designs, was enough to interest the most apathetic students in the mathematical ideas that the instructor was trying to impart.
Rollings, R. W. (2010). Polyhedra expressed through the beauty of wood, Journal of Mathematics and the Arts, 4(4), 191-199.
- In this article, the author demonstrated that how I have used the geometry of polyhedra and my chosen medium of lathe-turned wood to present the models shown in an aesthetically pleasing and artistic way.
- Theme: polyhedra was a focus of the author’s artistic works. The five platonic solids are the tetrahedron, hexahedron (cube), octahedron, dodecahedron and icosahedron. Each of the five platonic solids has been interpreted through the eyes of a wood turner, allowing the viewers alternate perspectives of these classic polyhedra.
Knoll, E. (2009). Pattern transference: making a ‘Nova Scotia tartan’ bracelet using the peyote stitch, Journal of Mathematics and the Arts, 3(4), 185-194.
- In this article, the author examines the parameters under which the Nova Scotia tartan can be transferred into an off-loom beading technique, known as peyote stitch, gourd stitch or twill stitch, by using the concepts from tiling theory, in order to produce a piece of wearable art.
- Theme: Despite the fact that twill weaves and peyote-stitched surfaces are the product of different techniques and are constructed in a very different sequence and manner, and since the degrees of freedom in design are not the same, the structural affinity derived from the topological equivalence between the homonymous techniques is made clear.
Sugihara, K. (2009). Computer-aided generation of Escher-like Sky and Water tiling patterns, Journal of Mathematics and the Arts, 3(4), 195-207.
- This article presents an interactive system for generating Escher-like Sky and Water tiling patterns. A typical example of such a print is Sky and Water I, in which a bird at the top deforms gradually towards the bottom and melts away into the background, while a fish gradually appears from the background.
- Theme: The authors have presented a [mathematical]method for generating Escher-like Sky and Water tiling patterns. In this method, the user supplies a pair of tiles, and the system automatically generates a tiling pattern in which one tile gradually melts away to the background while the other tile gradually appears from the background. The system combines tiling, morphing and figure-ground reversal. The user can also specify the density of and a global pattern for the tiling. This method therefore provides a computer-aided system that enables anyone to create Escher-like Sky and Water tiling patterns easily.
Harker, H. (2009). Group theory: students’ artistic visualizations, Journal of Mathematics and the Arts, 3(3), 119-122.
- Students in an introductory abstract algebra course often struggle with the basic concept of a group. The author presented an art project he assigned to his students to help address this challenge in which they were asked to visualize a group in an artistic medium of their choice.
- Theme: by creating a physical object they can identify with both a particular group and the mathematical idea of a group, each student built a memory to which they can attach the definition of a group. The author believes this memory along with the other students’ methods of visualizing different aspects of groups strengthened their connection to group theory.
Huber, M. R. (2009). The calculus of Gothic architecture, Journal of Mathematics and the Arts, 3(3), 147-153.
- What is seen in the Cathedral of Notre Dame in Paris can offer excellent examples of areas for students to calculate via integration. This article described an approach for instructors of single variable integral calculus courses in calculating the areas and volumes of Gothic structures.
- Theme: The rectangular towers, classical Gothic arches, massive domes and stained glass rose windows of this famous cathedral offer excellent examples of areas are good example to apply integration to calculate areas and volumes. In addition, these area calculations provide opportunities to apply trigonometric substitution in evaluating the area integrals.
Belcastro, S. M. (2009). Every topological surface can be knit: a proof, Journal of Mathematics and the Arts, 3(2), 67-83
- The authors developed a mathematical model for idealized knitting in terms of rubber-sheet topology.
- Theme: One goal of combining mathematics with knitting is to produce physical examples of mathematical objects. In this article it has showed that any topological surface can be knit with a single strand of yarn.
Williams, G. (2009). Using the Fractal Paintbrush, Journal of Mathematics and the Arts, 3(2), 85-96.
- This article explores some new methods for generating artwork with iterated functions systems, the computational tool central to the creation of many fractal images.
- Theme: In particular this article looked at the methods using lines or circles determined by points generated by an IFS (Iterated function system) system.
Akleman, E. (2009). Twirling sculptures, Journal of Mathematics, 3(1), 1-10.
- In this article, the author outlined a method for constructing aesthetically pleasing sculptures containing spiral shapes.
- Theme: with the help of computer graphics, many artists and scientists are now able to develop computational methods that use mathematics as a tool to create new aesthetic forms. Spirals are type of artistic examples which are popular in mathematics. Although spirals are usually represented by parametric equations, there are a wide variety of methods that can be used to construct and represent spirals. The author’s spiral construction arises from successive rotation and scaling of regular polygons in such a way that it resembles the construction of pursuit curves.
Whiteley, E. (2008). A process for generating 2D paintings and drawings from geometric diagrams, Journal of Mathematics and the Arts, 2(1), 29-38.
- The author used geometric composition figures themselves as the subject matter for artworks. He invented a generative process to transform 2D geometric figures representing root rectangles.
- Themes: Artworks can be created within the context of geometry. Root rectangles provide a fascinating starting point for a manual process for generating 2D paintings and drawings. The author began with a simple idea for transforming rectangles with the proportions of square roots and pursued it until he reached an aesthetic limit.
Stroud, J. B. (2008). Crockett Johnson’s geometric paintings, Journal of Mathematics and the Arts, 2(2), 77-99.
- This article is about Crockett Johnson’s abstract paintings. The paintings present gems in a large body of mathematical knowledge: without symbols, equations, or words, these works of art depict the often hidden beauty of mathematics.
- Theme: ‘Crockett Johnson’, this talented artist spent the last ten years of his life on an odyssey in mathematics, beginning with the study of historic mathematical milestones, including the three ‘impossible’ construction problems of the classical Greeks. While documenting in abstract paintings the achievements of others, he embarked on his own mathematical investigations, concluding his work with an original solution of the construction of a regular heptagon, using a technique employed by both Archimedes and Newton.
Frazier, L. &Schattschneider, D. (2008). Möbius bands of wood and alabaster, Journal of Mathematics and the Arts, 2(3), 107-122.
- Mo¨ bius bands, those mysterious one-sided surfaces, can be beautiful objects of art when carved as three-dimensional forms in exotic wood or alabaster. The author was writing his journey sculpting Mobius bands in the article.
- Theme: Mathematicians can rarely share the joy of their subject with non-mathematicians. But a Mo¨ bius strip, carved in richly-coloured wood with a wild swirly grain – everyone (well, almost everyone) can see the beauty in that.
Browne, C. (2008). Duotone Truchet-like tilings, Journal of Mathematics and the Arts, 2(4), 189-196.
- This paper explores methods for colouring Truchet-like tiles, with an emphasis on the resulting visual patterns and designs.
- Theme: The tilings can be made of different shapes like squares, triangles and hexagons. By different kinds of coloring like shadowing and coloring various corners of the shapes, a number of interesting geometrical patterns can be produced. This paper explores the visual opportunities offered by colourings of Truchet-like tilings that distinguish adjoining regions.
Browne, C. (2008). Duotone Truchet-like tilings, Journal of Mathematics and the Arts, 2:4, 189-196.
- A proof from plane geometry uses a diagram of a triangle, its altitudes, and three circles to prove the concurrency of the altitudes at the orthocentre. The proof characterizes the orthocentre as the radical centre of the three circles. The author showed that this diagram is also a self-sufficient device for drawing and analysing images in three-point perspective.
- Theme: By combining a particular geometry diagram and an unfolded box can create perspective drawings. by simply placing three faces of a given box on the diagram in a certain way, one can draw an accurate three-point perspective image of that particular box. Second, given a three-point perspective drawing or photograph of a box-shaped object, such as a package or a building, one can use the associated altitude diagram to determine the shape (relative proportions) of the original object. The diagram thus serves as a dual lesson in three-point perspective and plane geometry.
Gailiunas, P. (2007). Transformations – the sculpture of István Böszörményi, Journal of Mathematics and the Arts, 1(4), 225-233.
- This paper surveyed the geometric aspects of the Istva´n Boszormenyi’s work of sculpture, both the basic forms and how they were developed.
- Theme: Much of Istva´n Boszormenyi’s sculpture was based on simple geometric forms, which he modified in ways that were familiar to mathematicians, for example using affine transformations.
Hayes, L. D., (2007). Graph theory as a recurring theme in a series of mathematics and art course modules, Journal of Mathematics and the Arts, 1(4), 235-245.
- In this article Hayes (2007) presents a series of topics to be taught in a maths-art course (Celtic knots, Platonic solids, the fourth dimension, and the English art of change ringing), all of which require graph theory in a fundamental way.
- Theme: Some themes in mathematics, such as graph theory, can be taught in combined modules, such as math-art courses, as they are recurring in several mathematical subjects. Therefore, encouraging students of all levels to learn these themes through the math-art courses and activities help them learn the underlying mathematical concept better. In Hayes (2007), graph theory is the recurring theme that is suggested to be taught by various-edge colouring activities and knot construction through given instructions and some math.
- Nau, S., (2007). Artworks based on 2n = p + q, Journal of Mathematics and the Arts, 1(3), 191-201.
- Goldbach’s conjecture (any even number greater than 4 is expressible as the sum of two odd primes) has served as a springboard, providing Nau (2007) with inspiration for a prolonged series of artworks.
- Theme:This article is about creating artworks that can be produced based on the Goldbach’s Conjecture, e.g. 2n=p+q, where p and q are both add prime numbers. They look like piece-quilted blankets as the author, Nau (2007) sees them. The patterns produced that were inspired by this simple statement are tiled patterns with an even number of tiles that are partitioned into two sets. Each set consists of a prime number of tiles. These arrangements are cartoons, i.e. preparatory designs, drawings or paintings, used for the task of constructing aesthetically interesting artworks using traditional and non-traditional materials.
Ashlock, D., & Jamieson, B. (2007). Evolutionary computation to search Mandelbrot sets for aesthetic images, Journal of Mathematics and the Arts, 1(3), 147-158.
- Ashlock and Jamieson (2007) describe an evolutionary algorithm for searching Mandelbrot sets for aesthetic images.
- This article is proposing to use evolutionary algorithms to produce Mandelbrot sets and aesthetic images through designing fitness functions. Ashlock and Jamieson (2007) in this article apply the proposed technique to quadratic and cubic Mandelbrot sets that successfully appeared from their computer runs.
Mai, J. L., & Zielinski, D. (2007). Complete enumeration: a search for wholeness, Journal of Mathematics and the Arts, 1(3), 159-172.
- The authors examine, in six paintings spanning much of the artist’s career to date, how Mai has employed complete enumeration in a search for new visual forms.
- Theme: The article by Ami and Zielinski (2007) studies the mathematical foundations of the art of James Mai, an abstract painter. His art is considered as mathematical paintings that employ a range of simple relationships to complex combinations that further open new questions and mathematical sets. He explores complete enumeration structures in his art through methods such as systemic, permutational and self-revealing.
Friedman, N. (2007). Greg Johns: geometric sculpture, Journal of Mathematics and the Arts, 1(3), 173-180.
- The works of the Australian sculptor Greg Johns can be described as either figurative or geometric. Friedman (2007) concentrates on his geometric works that consist of curves in space.
- Theme: Friedman (2007) studies the art of an Australian sculptor, Greg Johns, and describe it as either figurative or geometric. In this paper he concentrates on the ones that consists of curves, including closed curves, open curves and knots and conclude that the sculptures convey a theme of strength which is due to the clean curving forms and well-proportionated square cross-sections.
Lee, S., Olsen, S., & Gooch, B. (2007). Simulating and analysing Jackson Pollock’s paintings, Journal of Mathematics and the Arts, 1(2), 73-83.
- The authors present an interactive system which allows users to create abstract paintings mimicking the style of Jackson Pollock using 3D viscous fluid jets.
- Theme: Lee, Olsen, and Gooch (2007) developed a system that let users use 3D viscous fluid jets that are guided by model simulations to create artworks similar to Jackson Pollock. His paintings include some fractal patterns that are modelled by computer to be used in the fluid simulator in the author’s experiment. Users can examine their Pollack-like paintings’ fractal properties and their aesthetic elements, mathematically, while they can only be recognized by human, subconsciously.
Fisher, G. L., & Mellor, B. (2007). Three-dimensional finite point groups and the symmetry of beaded beads, Journal of Mathematics and the Arts, 1(2), 85-96.
- In this article, Fisher and Mellor (2007) show that every finite subgroup of O(3) can be realized as the group of symmetries of a beaded bead through a weaving technique they developed themselves.
- Theme: Beaded Beads that are beads woven together are sometimes used to represent symmetries in three dimensional finite point groups, such as orthogonal group of degree three O(3). In this article, Fisher and Mellor (2007) show that every finite subgroup of O(3) can be realized as the group of symmetries of a beaded bead through a weaving technique they developed themselves. They also include examples of each subgroup of O(3) in their paper.
Dehlinger, H. (2007). On fine art and generative line drawings, Journal of Mathematics and the Arts, 1(2), 97-111.
- The algorithmic generation of line drawings is discussed from an artist’s point of view.zUsing artwork examples generated by the author, several different methods are considered and the underlying logic and algorithms are presented.
- Theme: In this paper Delinger(2007) have provided a descriptive view of his personal approach to generative line drawings. He has discussed and demonstrated the interdisciplinary thinking and processes that guide his algorithmic approach to art. He has given a detailed view of the mathematical processes, parameters and control mechanisms.
Demaine, E. D., Demaine, M.L., Taslakian, P., & Toussaint, G.T. (2007). Sand drawings and Gaussian graphs, Journal of Mathematics and the Arts, 1(2), 125-132.
- In this paper, we show connections between a special class of sand drawings and mathematical objects studied in the disciplines of graph theory and topology called Gaussian graphs.
- Theme: In this article a variety of basic questions about these ‘Gaussian graphs’are explored and it shows how these properties appear in real-life sand drawings. The connection between drawings and Gaussian graphs can help to understand the underlying mathematical structure of the drawings.
Xu, J., & Kaplan, C. S. (2007). Vortex maze construction, Journal of Mathematics and the Arts, 1(1), 7-20.
- Xu and Kaplan (2007) present an algorithm for producing mazes that they feel are difficult to solve. This algorithm is based on constructing and connecting obfuscating maze devices called vortices. A vortex is a collection of passages that wind around each other in a spiral and converge on a central junction. The authors explore variations on vortex construction that enable a range of aesthetic opportunities in the design of mazes and demonstrate our technique with several of our own examples.
- Theme: Amaze is simultaneously a mathematical abstraction, a work of art, and a window into the human mind. An effective maze is simultaneously a work of art and an engaging puzzle. The process of designing a maze must therefore balance the competing goals of complexity and aesthetics.
Maslanka, K. (2007). Polyaesthetics and mathematical poetry, Journal of Mathematics and the Arts, 1(1), 35-40.
- Polyaesthetics is a term Maslanka (2007) uses in connection with his artwork, as it embraces three different aesthetics; the aesthetics of verbal language, the aesthetics of visual language, and the aesthetics of mathematical language. His artwork can be regarded as a blend of ‘visual poetry’ and ‘mathematical poetry’. This paper focuses primarily upon the mathematical and verbal aspects of the author’s work, touching only briefly upon the visual aspects. It provides several examples to illustrate the process he uses to create polyaesthetic digital art based upon equations commonly encountered in physics and mathematics.
- Theme: Polyaesthetics connects the aesthetics of math, poetry and art by using each discipline in one context (the art work itself) to yield a rich aesthetic experience. Furthermore, mathematical poetry exemplifies the use of mathematical equations beyond science. One’s expression can range from the didactic to the spiritual to the aesthetics of ‘art for art sake’. The natural beauty of mathematics provides a limitless foundation to reflect the structure of the natural world and resonate within the personal structure of our belief systems and presents a source of inspiration to manifest a new-found synergetic aesthetic.
Sisson, P. D. (2007). Fractal art using variations on escape time algorithms in the complex plane, Journal of Mathematics and the Arts, 1(1), 41-45.
- This paper explores the use of orbit traps together with a simple escape time algorithm for creating fractal art based on nontrivial complex-valued functions. The purpose is to promote a more direct hands-on approach to exploring fractal artistic expressions.
- Theme: The creation of fractal art is easily accomplished through the use of widely-available software, and little mathematical knowledge is required for its use. But such an approach is inherently limiting and obscures many possible avenues of investigation. The article includes a gallery of images illustrating the visual effects of function, colour palette, and orbit trap choices.
Glaeser, G., & Gruber, F. (2007). Developable surfaces in contemporary architecture, Journal of Mathematics and the Arts, 1(1), 59-71.
- In this article the authors survey and discuss examples of the use of developable surfaces in contemporary architecture.
- Theme: Developable surfaces (tangential developables, in special cases cylinders and cones) are ruled surfaces with vanishing Gaussian curvature and can therefore be unfolded to the plane without distortions. These surfaces are a powerful tool for artists to use when designing architecture. Architectural designers are encouraged to directly design with developable surfaces instead of having to “break down” their prototypes later on. As a result, their designs will be easier (and cheaper) to build, since no triangulation will be necessary.
Barrallo, J., & Sanchez, S. (2001). Fractals and multi-layer coloring algorithm. In: Bridges Proceedings , (Winfield/USA), 89– 94.
- The focus of exploration in new fractal techniques is shifting away from fractal formulas and towards coloring algorithms. This paper provides an overview of the coloring algorithms in popular use and how they can be combined and superposed.
- Theme: A more significant technique involves combining techniques. The authors call these multi layer fractals, and they are the source of some of the richest fractal imagery being produced today. In this way, even a limited collection of fractal coloring algorithms can be combined in almost endless ways, with each combination becoming in effect an entirely new algorithm.
Fathauer, R.W. (2004). Fractal patterns and pseudo-tilings based on spirals. In: Bridges Proceedings, (Winfield/USA), 203–210.
- A variety of fractal patterns and pseudo-t Hings are described that are created by iterative arrangement of successively smaller spirals and spiral-shaped tiles. In some cases, these are used to create two-dimensional designs that resemble natural trees.
- Theme: The work presented here is by no means an exhaustive treatment of the possibilities for graphical fractal constructs based on spirals. Many different types of spirals, such as Fermat’s spiral or any of a variety of equiangular spirals weren’t explored at all. Countless different spiral prototiles could be constructed, and only a few of the possibilities for positioning smaller spirals relative to larger ones were explored here. It isn’t necessary for the spirals to be connected, nor is it necessary that they not be allowed to overlap. From an artistic perspective, there is a wide range of possibilities for rendering these constructions and for choosing settings in which to place them.
Cappellato, G., & Sala, N. (2017). Fractality in the arts and in architecture. Chaos and complexity letters, 11 (2), 239-265.
- The aim of this work is to describe as fractal geometry, its property of self-similarity, and its processes of bifurcation can be appeared in the arts, and in architecture.
- Theme: This paper has analyzed the interconnections between fractal geometry, arts and architecture. In particular the property of the self-similarity and the processes of bifurcation. Fractal geometry can help to introduce new paradigms in the arts and in architecture. Furthermore, the property of self-similarity of fractals can become a key to analyze the nature, the arts and architecture.
Mitchell, L.K. (2004). Fractal tessellations from proofs of the pythagorean theorem. In: Bridges Proceedings, (Winfield/USA), 335–336.
- The Pythagorean theorem can be proven geometrically through the use of dissections of squares and triangles. Four of these decompositions are paired into two compound dissections, which are used to create novel images.
- Theme: These compound dissections were used to create new artworks. These images merely hint at some of the possibilities open to the algorithmic artist. Of course, there are other ways to decompose squares and triangles into more squares and triangles, and each of those can be incorporated into a fractal tessellation. The varieties offered, combined with the freedom afforded by rotating the central square, make this technique quite a fruitful one.
Hamburger, P., & Hepp, E. (2005). Symmetric Venn Diagrams in the Plane: The Art of Assigning a Binary Bit String Code to Planar Regions Using Curves. Leonardo, 38(2), 125-132. Retrieved from http://www.jstor.org/stable/1577792
- The authors show how colors can be assigned to regions representing orbits of shifts of binary strings, thus creating unusual images.
- Theme: The authors discuss artwork created by assigning a binary string code with length 11 each of 211 = 2,048 planar regions formed by the intersection of 11 rotations of a single simple closed curve over 360/11 degrees. The goal of this process is to create the maximum number of connected regions, exactly one for each the 2,048 different binary strings with length 11.
Zielinski, D. (2006). Counting on the Art of James Mai. Math Horizons, 13(3), 18-21. Retrieved from http://www.jstor.org/stable/25678600
- Professor and artist James Mai uses organizations of geometric shapes and color to create paintings that invite the viewer to discover the general ordering principle of each work of art.
- Theme: Number theory, combinatorics, figurate numbers and graph theory are mathematical elements that Mai apply in his art works.
Mai, J.L. & Zielinski, D. (2006). Constellations of form: new compositional elements related to polyominoes. proceedings of Bridges London 2006: Mathematics, Music, Art, Architecture, Culture, London Knowledge Lab, Institute of Education, University of London, London, UK, 351–358.
- The creative process of the artist, James Mai, and an exploration of the mathematics within Permutations are the subjects of this paper.
- A mathematical analysis using Burnside’s Theorem provided by Daylene Zielinski lays out an orderly approach that can be implemented in this type of artistic investigation. This useful theorem can tell the artist, in advance, how many distinct forms he will discover. It is important to note, however, that Burnside’s Theorem says nothing about what these forms will look like or how to find them all; it predicts only the final count of visually distinct figures which can be created under a set of rules determined by the artist.
Mai, J.L. & Zielinski, D. (2006). Constellations of form: new compositional elements related to polyominoes. Proceedings of Bridges London: Mathematics, Music, Art, Architecture, Culture, London Knowledge Lab, Institute of Education, University of London, London, UK, 351–358.
- This article study Mai’s artworks related to permutational process.
- Theme: A predominant theme of artist James Mai’s compositions is the development of finite sets of related objects derived from permutational processes. Each element is distinct, yet all of them share particular features. Thus, he develops families of objects that are at once diverse since each object is visually distinct and integral since the set of objects is exhaustive.
Mitani, J. & Suzuki, H. (2004). Making papercraft toys from meshes using strip-based approximate unfolding. ACM Transactions on Graphics, 23(3), 259–263.
- Mitani and Suzuki (2004) proposed a new method for producing unfolded papercraft patterns from triangulated meshes by means of strip-based approximation.
- Theme: Mitani and Suzuki (2004) propose a new method for producing unfolded papercraft patterns of rounded toy animal figures from triangulated meshes by means of strip-based approximation. The distinguishing feature of our method is that we approximate a mesh model by a set of continuous strips, not by other ruled surfaces such as parts of cones or cylinders. Thus, the approximated unfolded pattern can be generated using only mesh operations and a simple unfolding algorithm.
Bonner, J. (2003). Three Traditions of Self-Similarity in Fourteenth and Fifteenth Century Islamic Geometric Ornament, Isama -Bridge Conference Proceedings, Granada, Spain, 1- 12.
- The traditional techniques used in the creation of Islamic self-similar patterns are overviewed in this article.
- Theme: In both the western and the eastern regions during the fourteenth and fifteenth centuries respectively, previously established systematic methods of two-dimensional geometric pattern construction were used in the development of three distinct traditions of self-similar geometric design. These innovations resulted in the last great advancement in the long history of Islamic geometric pattern making. Furthermore, these patterns are very likely the first, and among the most engaging, examples of complex overtly self-similar art made by man.
Gerdes, P. (1989). Reconstruction and extension of lost symmetries: Examples from Tamil of South India, Comp. Math. Applic, 17(4–6), 791–813.
- The analysis of the article reveals the high mathematical potential of the tradition that led to the Tamil threshold designs.
- Theme: Many traditional Tamil threshold designs are made out of one closed, smooth fine. An investigation of Tamil patterns which do not conform to their cultural standard as they are composed of two, three or more superimposed closed paths, shows that these designs are “degraded” versions of originally more symmetrical patterns made out of single “never-ending” lines.
Bapat, M. (2008). Mathematics in RangoLee art from India. Proceedings of Bridges, ( Leeuwarden/The Netherland), 429-432.
- In this paper the author categorizes rangolee (ancient art of floor decoration from India, built on rectangular or hexagonal arrays of dots which serve as base for designs and patterns based on abstractions from the natural/cultural world designs) according to the method by which they are created and the motives that appear in them.
- Theme: The author links the traditional and contemporary designs with patterns and models that have arisen independently in the world of mathematics. This includes a variety of symmetry groups, spirals, mirror curves, fractal self-similarity, and processes of iteration.
Sala, N. (2006). Fractal geometry and architecture: Some interesting connections. WIT Transaction on The Built Environment, 11, 163-173.
- The aim of this paper is to present a fractal analysis applied to different architectural styles.
- Theme: In architectural design is important to provide harmony between old and new. Fractal geometry can be used in the process of supporting creativity in the ideation of new forms and for testing harmony between old and new.
Piro, T. D. (2008). Cosmati pavements: The art of geometry, in Proceedings of Bridges Leeuwarden, Reza Sarhangi and Carlo Se´quin, eds., Tarquin Books, London, 369–376.
- This paper considers some pavement designs of the group of artists known as “The Cosmati”. The authors argue that their designs are primarily concerned with conveying the aesthetic of beauty, and yet still have deep implications in the study of geometry.
- Theme: The effect of aesthetics on geometry is, primarily, to find new methods of representing curves either in a plane or in 3-dimensional space (for this group of artists). There are four aesthetic categories can be and has been used in the study of algebraic curves by these artists:1. The aesthetic of fragmentation and linearity, 2. The aesthetic of light, 3. The aesthetic of harmony, 4. The aesthetics of fluency, dynamism and spiraling have a geometric interpretation in the case of real plane analytic curves.
Johnson, C. (1972). On the mathematics of geometry in my abstract paintings. Leonardo, 5 (2), 97-101.The MIT Press. Retrieved August 8, 2018, from Project MUSE database.
- The artist, who is not a mathematician, describes his use of geometry as an artist’s tool in his painting.
- Theme: In recent times, art has echoed the language of geometry with terms like ‘the golden mean canvas’, ‘dynamic symmetry’, ‘cubism’ and ‘geometric’, all of which have had little relation to the geometry of the mathematicians. In this article the author explains how he uses, as intrinsic tools, the mathematical geometry and mathematical methods in his geometrical painting.
Emmer, M. (1980). Visual art and mathematics: The Moebius band, Leonardo, 13(2), 108-111.
- The author describes briefly the mathematical properties of the Moebius band and its use in the works of several other artists.
- Theme: The purpose of the article is to draw attention to the way a simple topological form has been arrived at independently by a mathematician and by a visual artist and to how some artists have reacted to it by incorporating it in their works.
Bapat, M. (2015). Use of rangolee art in elementary mathematics education. Proceeding of Bridges.
- In this workshop paper a strategy to teach basic and advanced math concepts using RangoLee designs is discussed.
- Theme: There are four basic mathematical principles in RangoLee designs: algorithmic thinking, Eulerian graphs, transformational geometry, and iteration. In some RangoLee designs one may find reflection across an axis (mirror symmetry). Some other designs show rotation around a single point. Finally, in many south Indian designs one finds scaling symmetry applied in cycles: you can build bigger patterns out of a smaller base pattern. Mathematicians identify this type of iteration as a recursion. The iterations allow the implementation of many NCTM algebra standards along with number work, math facts, symmetry, geometry, measurement, as well as making connections between math and other subjects such as art and culture.
Hill, A. (1977). A View of Non-figurative Art and Mathematics and an Analysis of a Structural Relief. Leonardo 10(1), 7-12. The MIT Press. Retrieved August 8, 2018, from Project MUSE database.
- The author discusses some interpretations of the term mathematics and what can be meant by mathematical abstract or non-figurative visual art.
- Theme: In this article the author discusses the meaning of a kind of non-figurative or ‘abstract’ visual art that can be described as being, in part, ‘mathematical’. This property is not to be taken as a physical characteristic or parameter of this art, such as the dimensions of length, time and mass of different kinds of art media. However, a work of art classified as ‘mathematical’ is one in which some aspects of its organization can be shown to involve the manipulation of concepts or ideas that, at least in part, stem from those found in some branch of mathematics.
Hamburger, P. (2002). Doilies and Doodles, Non-Simple Symmetric Venn Diagrams. Discrete Mathematics, (2-3), 423-439.
- In this article the author creates a method to construct a non-simple 11-doily.
- Theme: This technique does not use any computer search. The real difficulty here is to show a decent drawing of such diagrams in a paper of limited size.
Demaine, E. D., Gomez-Martin, F., Meijer, H., Rappaport, D., Taslakian, P., Toussaint, G. T., Terry Winograd, T., & Wood, D. R. (2009). The distance geometry of music. Computational Geometry: Theory and Application, 42(5),429–454.
- The authors demonstrate relationships between the classic Euclidean algorithm and many other fields of study, particularly in the context of music and distance geometry.
- Theme: The authors show how the structure of the Euclidean algorithm defines a family of rhythms which encompass over forty timelines (ostinatos) from traditional world music. The authors prove that these Euclidean rhythms have the mathematical property that their onset patterns are distributed as evenly as possible: they maximize the sum of the Euclidean distances between all pairs of onsets, viewing onsets as points on a circle. Indeed, Euclidean rhythms are the unique rhythms that maximize this notion of evenness.
Castro, M., & Pérez-Luque, M. (2003). Fractal geometry describes the beauty of infinity in nature. In: Bridges Proceedings, 407–414.
- The article explains the foundations of the natural law which has expressed the infinite through history, and its attempt to reach it as beauty expression, to conclude with a proposal to define a new way to achieve it, through fractal geometry and new technologies.
- Theme: The authors through the bionic science· and the fractal geometry, have discovered the expression of infinite as the internal natural order. This order is what the authors call beauty. And beauty has been the desire of art along history. That order, beauty, is the same law that nature uses to achieve optimal system structure solutions. As the result to design using that order, natural, optimal, environmental and beautiful artifacts are achieved.
Adams, K. R. (1972). Perspective and the viewpoint. Leonardo, 5(3), 209-217.
- In this article the geometrical principle of perspective and the viewpoint are explained. [This discussion can be useful for painters, so I chose this article].
- Theme: Geometrical perspective can provide a precise set of rules for the interpretation of a pattern of marks on a plane as a pattern of things in space. A viewer whose vision conforms to these rules will be highly responsive to the picture plane relative to his exact viewpoint.
Greene, R. (1983). Determining the Preferred Viewpoint in Linear Perspective. Leonardo, 16(2), 97-102.
- In this article the basic theory of linear perspective is reviewed. [This discussion can be useful for painters, so I chose this article].
- Theme: It is found that the horizon in a picture can give the vertical position of the viewpoint. The outside knowledge or assumptions which are necessary in order completely to determine the preferred viewpoint are then considered. It is found that a three-point perspective provides more information about the viewpoint.
Langae, A., & Dutre, P. (2006). An alternative for Wang tiles: Colored edges versus colored corners. ACM Transactions on Graphics, 25(4), 1442-1459.
- In this article the authors propose the benefits of corner tiles compared with the Wang tiles. Important applications of Wang tiles include texture synthesis, tilebased texture mapping, and generating Poisson disk distributions.
- Theme: Compared to Wang tiles (Wang tiles are square tiles with colored edges, placed randomly next to each other such that adjoining edges have matching colors) corner tiles are easier to tile, textures synthesized with corner tiles contain more samples from the original texture, corner tiles reduce the required texture memory by a factor of two for tile-based texture mapping, and Poisson disk distributions generated with corner tiles have better spectral properties. Corner tiles result in cleaner, simpler, and more efficient applications.